Basics of Waves Review
Navigate to:
Review Session Home - Topic Listing
Waves - Home || Printable Version || Questions with Links
Answers to Questions: All || #1-#14 || #15-#26 || #27-#38
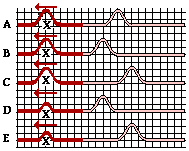
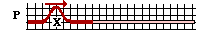27. Diagram P at the right shows a transverse pulse traveling along a dense rope toward its junction with a less dense rope. Which of the diagrams (A, B, C, D, or E) below depicts the ropes at the instant that the reflected pulse again passes through its original position marked X? Consider such features as amplitude and relative speed (i.e., the relative distance of the transmitted and reflected pulses from boundary).
|
Answer: E
A, B, and C can be quickly ruled out since it shows the amplitude of the reflected and incident pulse to be the same size. An incident pulse would give up some of its energy to the transmitted pulse at the boundary, thus making the amplitude of the reflected pulse less than that of the incident pulse. Rule out D since it shows the reflected pulse moving faster than the transmitted pulse. This would not happen unless moving from less dense to more dense. This leaves E as the answer.
|
[ #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 ]
28. A wave whose speed in a snakey is 4.4 m/s enters a second snakey. The wavelength changes from 2.0 m to 3.0 m. The wave in the second snakey travels at approximately ____.
|
a. 1.5 m/s.
|
b. 2.2 m/s.
|
c. 2.9 m/s.
|
d. 4.4 m/s.
|
e. 6.6 m/s.
|
|
Answer: E
This is another boundary behavior question with a mathematical slant to it. The frequency of the incident and transmitted waves are always the same. Thus, use f =v/w to find the frequency of the incident wave - 2.2 Hz. The frequency of the transmitted wave is >also 2.2 Hz, the wavelength is 3.0 m, and so the speed is f*w = 6.6 m/s.
|
[ #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 ]
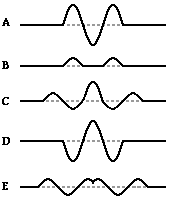
2
9. The diagram at the right shows a disturbance moving through a rope towards the right. If this disturbance meets a similar disturbance moving to the left, then which one of the diagrams below depict a pattern which could NEVER appear in the rope?
|
Answer: D
WOW! Tough one. Draw a second wave to the right of the wave which is given. Then visually move the wave to the left. Visualize in your mind the shape of the resultant as interference occurs. It will never look like D. If you still don't get it, take a break and watch some TV.
|
[ #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 ]
30. A 2.0-meter long rope is hanging vertically from the ceiling and attached to a vibrator. A single pulse is observed to travel to the end of the rope in 0.50 s. What frequency should be used by the vibrator to maintain three whole waves in the rope?
|
a. 0.75 Hz
|
b. 1.3 Hz
|
c. 4.0 Hz
|
d. 6.0 Hz
|
e. 8.0 Hz
|
|
Answer: D
The given info allows you to determine the speed of the wave: v=d/t=2 m/0.5 s) = 4 m/s. If there are 3 waves in a 2-meter long rope, then each wave is 2/3-meter long. Now find frequency with the equation v=f*w where v=4 m/s and w=0.667 m. Proper algebra yields 6 Hz as the answer.
|
[ #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 ]
31. A standing wave experiment is performed to determine the speed of waves in a rope. The standing wave pattern shown below is established in the rope. The rope makes exactly 90 complete vibrational cycles in one minute. The speed of the waves is ____ m/s.
|
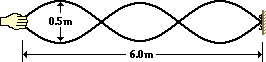
a. 3.0
|
b. 6.0
|
c. 180
|
d. 360
|
e. 540
|
|
Answer: B
If there are exactly 90 vibrations in 60.0 seconds, then there is a frequency of 1.5 Hz. The diagram shows 1.5 waves in 6.0-meters of rope; thus, the wavelength is 4.0 meters. Now use the equation v=f*w to calculate the speed of the wave. Proper substitution yields 6.0 m/s.
|
[ #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 ]
32. Consider the standing wave pattern shown below. A wave generated at the left end of the medium undergoes reflection at the fixed end on the right side of the medium. The number of antinodes in the diagram is _____.
|
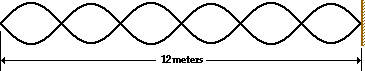
a. 3
|
b. 5
|
c. 6
|
d. 7
|
e. 12
|
|
Answer: C
An antinode is a point on the medium which oscillates from a large + to a large - displacement. Count the number of these points - there are 6 - but do not count them twice.
|
[ #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 ]
33. A node is a point located along the medium where there is always ___.
|
a. a double crest
|
b. a double trough
|
c. constructive interference
|
|
d. destructive interference
|
e. a double rarefaction
|
|
|
Answer: D
A node is a point along the medium of no displacement. The point is not displaced because destructive interference occurs at this point.
|
[ #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 ]
34. TRUE or FALSE:
Constructive interference of waves occurs when two crests meet.
|
Answer: A
Yes! Or when a trough meets a trough or whenever two waves displaced in the same direction (such as both up or both down) meet.
|
[ #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 ]
35. Which phenomenon is produced when two or more waves passing simultaneously through the same medium meet up with one another?
|
a. refraction
|
b. diffraction
|
c. interference
|
d. reflection
|
|
Answer: C
Interference is the meeting of two or more waves when passing along the same medium - a basic definition which you should know and be able to apply.
|
36. Two pulses are traveling in opposite directions along the same medium as shown in the diagram at the right. Which diagram below best depicts the appearance of the medium when each pulse meets in the middle?
|
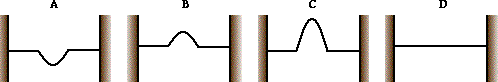
Answer: D
When a crest is completely overlapped with a trough having the same amplitude, destructive interference occurs. Complete cancellation takes place if they have the same shape and are completely overlapped.
|
[ #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 ]
37. TRUE or FALSE:
A vibrating object is necessary for the production of sound.
|
Answer: A
Absolutely! If you don't believe it, then think of some sounds - voice, guitar, piano, tuning fork, chalkboard screech, etc. - and consider what the vibrational source is. All sounds have a vibrating object of some kind as their source.
|
[ #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 ]
38. Which one of the following CANNOT transmit sound?
|
a. Liquid air
|
b. Gaseous oxygen
|
c. Liquid water
|
|
d. Solid steel
|
e. Perfect vacuum
|
|
|
Answer: E
Sound is a mechanical wave and as such requires a medium in order to move through space. For this reason, sound cannot move through a vacuum.
|
[ #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 ]
Navigate to:
Review Session Home - Topic Listing
Waves - Home || Printable Version || Questions with Links
Answers to Questions: All || #1-#14 || #15-#26 || #27-#38
You Might Also Like ...
Users of The Review Session are often looking for learning resources that provide them with practice and review opportunities that include built-in feedback and instruction. If that is what you're looking for, then you might also like the following:
- The Calculator Pad
The Calculator Pad includes physics word problems organized by topic. Each problem is accompanied by a pop-up answer and an audio file that explains the details of how to approach and solve the problem. It's a perfect resource for those wishing to improve their problem-solving skills.
Visit: The Calculator Pad Home | Calculator Pad - Vibrations and Waves
- Minds On Physics the App Series
Minds On Physics the App ("MOP the App") is a series of interactive questioning modules for the student that is serious about improving their conceptual understanding of physics. Each module of the series covers a different topic and is further broken down into sub-topics. A "MOP experience" will provide a learner with challenging questions, feedback, and question-specific help in the context of a game-like environment. It is available for phones, tablets, Chromebooks, and Macintosh computers. It's a perfect resource for those wishing to refine their conceptual reasoning abilities. Part 5 of the series includes topics on Wave Motion.
Visit: MOP the App Home || MOP the App - Part 5