Reflection and Mirrors Review
Navigate to:
Review Session Home - Topic Listing
Reflection and Mirrors - Home || Printable Version || Questions with Links
Answers to Questions: All || #1-#19 || #20-#26 || #27-#32 || #33-#42
Part A: Multiple Choice
1. As the angle of incidence is increased for a ray incident on a reflecting surface, the angle between the incident and reflected rays ultimately approaches what value?
|
a. zero
|
b. 45 degrees
|
c. 90 degrees
|
d. 180 degrees
|
|
Answer: D
The angle of incidence is the angle between the incident ray and the normal. As this angle approaches 90 degrees, the reflected ray also approaches a 90 degree angle with the normal; thus, the angle between the incident and reflected ray approach 180 degrees.
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
2. If you stand three feet in front of a plane mirror, how far away would you see yourself in the mirror?
|
a. 1.5 ft
|
b. 3.0 ft
|
c. 6.0 ft
|
d. 12.0 ft
|
|
Answer: C
If you stand 3 feet from the mirror, then your image is three feet on the other side of the mirror; this puts your image a total of six feet from you (3 feet to the mirror plus 3 more feet to the image).
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
3. A concave mirror with a focal length of 10.0 cm creates a real image 30.0 cm away on its principal axis; the corresponding object is located how far from the mirror?
|
a. 20.0 cm
|
b. 15.0 cm
|
c. 7.5 cm
|
d. 5.0 cm
|
|
Answer: B
Use the mirror equation:
1/di + 1/do = 1/f
where di = +30 cm and f = +10 cm.
Substitute and solve for do.
1/do + 1/(30 cm) = 1/(10 cm)
1/do = 1/(10 cm) - 1/(30 cm) = 2/(30 cm)
do = 15 cm
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
4. A concave mirror forms a real image at 25.0 cm from the mirror surface along the principal axis. If the corresponding object is at a 10.0 cm distance, what is the mirror's focal length?
|
a. 1.4 cm
|
b. 16.7 cm
|
c. 12.4 cm
|
d. 7.1 cm
|
|
Answer: 7.1 cm
Use the mirror equation:
1/di + 1/do = 1/f
where di = +25 cm and do = +10 cm.
Substitute and solve for f.
1/(10 cm) + 1/(25 cm) = 1/f
1/f = 14/(100 cm) or 0.14/cm
f = 100/14 cm = 7.1 cm
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
5. If a virtual image is formed along the principal axis 10.0 cm from a concave mirror with the focal length 15.0 cm, what is the object distance from the mirror?
|
a. 30.0 cm
|
b. 10.0 cm
|
c. 12.4 cm
|
d. 6.0 cm
|
|
Answer: D
Use the mirror equation:
1/di + 1/do = 1/f
where di = -10 cm and f = +15 cm. (Note that di is negative if the image is virtual.)
Substitute and solve for do.
1/do + 1/(-10 cm) = 1/(15 cm)
1/do = 1/(15 cm) - 1/(-10 cm) = 5/(30 cm)
do = 6 cm
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
6. If a virtual image is formed 10.0 cm along the principal axis from a convex mirror of focal length -15.0 cm, what is the object distance from the mirror?
|
a. 30.0 cm
|
b. 10.0 cm
|
c. 6.0 cm
|
d. 3.0 cm
|
|
Answer: A
Use the mirror equation:
1/di + 1/do = 1/f
where di = -10 cm and f = -15 cm. (Note that di is negative if the image is virtual.)
Substitute and solve for do.
1/do + 1/(-10 cm) = 1/(-15 cm)
1/do = 1/(-15 cm) - 1/(-10 cm) = -1/(30 cm)
do = 30 cm
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
7. If a man's face is 30.0 cm in front of a concave shaving mirror creating an upright image 1.50 times as large as the object, what is the mirror's focal length?
|
a. 12.0 cm
|
b. 20.0 cm
|
c. 70.0 cm
|
d. 90.0 cm
|
|
Answer: D
First find di.
If the do = 30 cm and the M = +1.5 (Note: M is + since the image is upright), then use M = -di/do to find that di = -45 cm (the - di value is consistent with the image being virtual - located behind the mirror).
Now use the mirror equation to find the focal length:
1/di + 1/do = 1/f
where di = -45 cm and do = +30 cm.
Substitute and solve for f.
1/(30 cm) + 1/(-45 cm) = 1/f
1/f = 1/(90 cm) or 0.011/cm
f = 90 cm
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
8. Which of the following best describes the image formed by a plane mirror?
- virtual, inverted and enlarged
- real, inverted and reduced
- virtual, upright and the same size as object
- real, upright and the same size as object
|
Answer: C
When you look at your image in a plane mirror, you see an upright image; it is located on the other side of the mirror (and thus is virtual); finally, it has the same dimensions (height, width) as yourself (the object).
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
9. Which of the following best describes the image formed by a concave mirror when the object is located somewhere between the focal point (F) and the center of curvature (C) of the mirror?
|
a. virtual, upright and enlarged
|
b. real, inverted and reduced
|
|
c. virtual, upright and reduced
|
d. real, inverted and enlarged
|
|
Answer: D
For concave mirrors, when the object is located anywhere between F and C, the image is real, inverted, enlarged in size, and located beyond C. You should get this very result if you were to draw a ray diagram.
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
10. Which of the following best describes the image formed by a concave mirror when the object is at a distance further than the center of curvature (C) of the mirror?
|
a. virtual, erect and enlarged
|
b. real, inverted and reduced
|
|
c. virtual, upright and reduced
|
d. real, inverted and enlarged
|
|
Answer: B
For concave mirrors, when the object is located anywhere beyond C, the image is real, inverted, reduced in size, and located between F and C. You should get this very result if you were to draw a ray diagram.
|
[ #1| #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
11. Which of the following best describes the image formed by a concave mirror when the object distance from the mirror is less than the focal length (f)?
|
a. virtual, upright and enlarged
|
b. real, inverted and reduced
|
|
c. virtual, upright and reduced
|
|
|
Answer: A
For concave mirrors, when the object is located anywhere inside the F, the image is virtual, upright, enlarged in size, and located on the opposite side of the mirror. You should get this very result if you were to draw a ray diagram.
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
12. Which of the following best describes the image formed by a convex mirror when the object distance from the mirror is less than the absolute value of the focal length (f)?
|
a. virtual, upright and enlarged
|
b. real, inverted and reduced
|
|
c. virtual, upright and reduced
|
d. real, inverted and enlarged
|
|
Answer: C
For convex mirrors, regardless of where the object is located, the image is virtual, upright, reduced in size, and located on the opposite side of the mirror. You should get this very result if you were to draw a ray diagram.
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
13. Use of a parabolic mirror, instead of one made of a circular arc surface, can be used to reduce the occurrence of which of the following effects?
|
a. spherical aberration
|
b. mirages
|
|
c. chromatic aberration
|
d. light scattering
|
|
Answer: A
The problem of spherical aberration are caused by the inability of the outer portions of a concave spherical mirror to reflect and focus light to the same image locations as other portions of the mirror. A cure for the problem involves the use of a mirror with a different shape in the regions far from the principal axis; a parabolic mirror solves this problem.
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
14. When the image of an object is seen in a plane mirror, the image is _____.
|
a. real and upright.
|
b. real and inverted.
|
|
c. virtual and upright.
|
d. virtual and inverted.
|
|
Answer: C
Look at yourself in a plane mirror and you see your image - it is upright. The image is located on the other side of the mirror since reflected rays diverge upon reflection; when mirrors produce images on the the opposite side of the mirror, the images are said to be virtual.
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
15. When the image of an object is seen in a plane mirror, the distance from the mirror to the image depends on _____.
- the wavelength of light used for viewing.
- the distance from the object to the mirror.
- the distance of both the observer and the object to the mirror.
|
Answer: B
For plane mirrors, the image distance is the same as the object distance (di =-do). The only way to modify the image distance is to modify the object distance.
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
16. If a man wishes to use a plane mirror on a wall to view both his head and his feet as he stands in front of the mirror, the required length of the mirror _____.
- is equal to the height of the man.
- is equal to one half the height of the man.
- depends on the distance the man stands from the mirror.
- depends on both the height of the man and the distance from the man to the mirror.
|
Answer: B
The portion of mirror required to view the full image of an object is always one-half the height of the object (for plane mirrors only). For the skeptics who believe C is the more believable answer, see the proof given on the Physics Classroom tutorial page.
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
17. When the image of an object is seen in a concave mirror the image will _____.
|
a. always be real.
|
b. always be virtual.
|
|
c. be either real or virtual.
|
d. will always be magnified.
|
|
Answer: C
Concave mirrors can produce real images (if the object is beyond the focal point) and virtual images (if the object is located in front of the focal point). These images can be either magnified in size (if object is in front of F or between C and F), reduced in size (if object is located beyond C),or the same size as the object (if object is located at C).
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
18. When the image of an object is seen in a convex mirror the image will _____.
|
a. always be real.
|
b. always be virtual.
|
|
c. may be either real or virtual.
|
d. will always be magnified.
|
|
Answer: B
Convex mirrors always (always) produce images which are virtual, upright, and reduced in size. Since reflected light rays always diverge from each other, these convex mirrors can never produce real images. Draw a ray diagram to convince yourself that this is true.
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
19. Rays of light traveling parallel to the principal axis of a concave mirror will come together _____.
|
a. at the center of curvature.
|
b. at the focal point.
|
|
c. at infinity.
|
d. at a point half way to the focal point.
|
|
Answer: B
The focal point is the location where light rays traveling parallel to the principal axis converge. (Perhaps you remember the demo with the concave (converging) mirror and the pencil or dollar bill.)
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
20. Right angle mirrors produce three images of objects. Which of the following is statements is/are true of the middle image?
- The middle image is the same size as the object.
- The middle image is the same distance from the mirror as the object.
- The middle image will exhibit left-right reversal relative to the object.
- The middle image is a real image.
- The middle image is an upright image.
- The magnification of the middle image is -1.
- When sighting at the middle image, light will reflect twice prior to reaching one's eye.
|
Answer: ABEG
A is true; like any plane mirror image, the image formed by two mirrors oriented at right angles is the same size as the object.
B is true; the middle image is the same distance from the crease of the dual mirror system as the image is from the crease. However, image distances are usually measured as the smallest distance measured perpendicularly to the mirror. For middle images, this distance would be measured to an extended mirror line and it would be the same distance as that from the object to that same mirror.
C is false; the middle image, unlike the primary images, does not exhibit this feature of left-right reversal.
D is false; the middle image is a virtual image; it is upright and located behind the mirror (like any virtual image).
E is true; just look at the middle image and you will see an upright image.
F is false; a magnification of -1 means that the image would be inverted (negative M) and the same size as the object (1); the middle image is the same size as the object but it is not inverted.
G is true; light will reflect twice - once off each mirror - prior to traveling to the viewer's eye.
|
21. Two plane mirrors are attached to form a dual mirror system with an adjustable angle. As the angle between the mirrors increases, the number of images _____.
|
a. increase
|
b. decreases
|
c. remains the same
|
|
Answer: B
Consider two mirrors arranged at a 0-degree angle - parallel to each other. There would be an infinite number of images, one located directly behind the other forming a line which seems to extend forever. Suppose the mirrors are adjusted to a 30-degree angle (see question #29 below). The mirrors would create 5 images of objects placed between the mirrors. Now suppose that the angle is increased to a 90-degree angle (see question #29 below). Finally, suppose the mirrors are adjusted to a 180-degree angle to form a single plane. At such an angle, only one image would be formed. As the angle is increased, the number of images decreases.
|
22. Which of the following statements is/are true of a virtual image?
- Virtual images are always located behind the mirror.
- Virtual images can be either upright or inverted.
- Virtual images can be magnified in size, reduced in size or the same size as the object.
- Virtual images can be formed by concave, convex and plane mirrors.
- Virtual images are not real; thus you could never see them when sighting in a mirror.
- Virtual images result when the reflected light rays diverge.
- Virtual images can be projected onto a sheet of paper.
|
Answer: ACDF
A is true; virtual images are always located behind the mirror.
B is false; virtual images are always upright.
C is true; a virtual image is reduced in size if formed by a convex mirror; it is magnified in size when created by concave mirrors; it is the same size as the object when created by plane mirrors.
D is true; convex and plane mirrors always form virtual images; concave mirrors will form virtual images of objects placed between the focal point and the mirror.
E is false; while virtual images are not real, they can be seen if sighted at in the mirror - just consider your plane mirror image which you probably view everyday.
F is true. Virtual images result when incident light from the object strikes the mirror, reflects and diverges. The virtual image is located behind the mirror at the spot where the reflected rays would converge if extended behind the mirror.
G is false; only real images which are formed in front of mirrors can be projected onto the screen. Virtual images are located behind the mirror where the light does not travel.
|
23. Which of the following statements is/are true of a real image?
- Real images are always located behind the mirror.
- Real images can be either upright or inverted.
- Real images can be magnified in size, reduced in size or the same size as the object.
- Real images can be formed by concave, convex and plane mirrors.
- Real images are not virtual; thus you could never see them when sighting in a mirror.
- Real images result when the reflected light rays diverge.
- Real images can be projected onto a sheet of paper.
|
Answer: CG
A is false; real images are always located in front of the mirror.
B is false; real images are always inverted and never upright.
C is true; a real image can be magnified if placed between C and F of a concave mirror; it can be reduced in size if placed beyond C in front of a concave mirror; and it can be the same size as the object if placed at C in front of a concave mirror.
D is false; convex and plane mirrors always form virtual images and can never form real images; only a concave mirror can form both types of images.
E is false; real images can be seen when sighting at the image location (just like virtual images can).
F is false; real images result when reflected light rays converge to a point.
G is true; real images (unlike virtual images) can be projected onto a sheet of paper. Because real images form in front of the mirror at the locations where reflected rays converge, the image can be projected onto a sheet of paper if it is placed at this image location.
|
24. Which of the following situations would always result in an image which is magnified in size?
- Concave mirror: di > 0 and do < di
- Convex mirror: do > 0
- Plane Mirror: do > 0
- Concave Mirror: do> R
- Concave Mirror: do< f
- Concave Mirror: do> f and do < R
|
Answer: AEF
A results in a magnified image. If the image distance is greater than 0, then the image is a real image. If the image distance is greater than the object distance, then according to the magnification ratio, the image height must also be equal to the object height.
In B and C, a convex and a plane mirror never creates a magnified image. Convex mirrors always create images which are reduced in size. Plane mirrors always create images which are the same size as the object.
In D, the object is placed beyond the center of curvature of a concave mirror. In such an instance, the image is reduced in size and located between C and F.
In E, the object is placed between the focal point and a concave mirror. At such an object location, the image is virtual, magnified, and upright.
In F, the object is placed in between points C and F of a concave mirror. For such an object location, the image is real, magnified, and located beyond the center of curvature.
|
25. Which of the following situations would always result in an image which is upright?
- Concave mirror: di > 0 and do < di
- Convex mirror: do > 0
- Plane Mirror: do > 0
- Concave Mirror: do> R
- Concave Mirror: do< f
- Concave Mirror: do> f and do < R
|
Answer: BCE
In A, the image is real and its distance is further from the mirror than the object. This could only occur when the object is placed between C and F of a concave mirror. For such an object location, the image is real, inverted, and magnified in size.
In B, a convex mirror is used. Convex mirrors always produce upright, virtual images.
In C, a plane mirror is used. Plane mirrors always produce upright, virtual images.
In D, the object is located beyond the center of curvature of a concave mirror. At such an object location, the image would be real, inverted and reduced in size.
In E, the object is located in front of the focal point of a concave mirror. At such an object location, the image would be virtual, upright, and magnified.
In F, the object is located between the center of curvature and the focal point of a concave mirror; the image would be real, inverted, and magnified.
|
26. TRUE or FALSE:
Virtual images formed by mirrors are always upright images.
|
Answer: A
This is a true statement. The image characteristics of being upright and being virtual go hand in hand. If the image is virtual, then it is upright; and vice versa. Only real images are inverted and only inverted images are real.
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
Part B: Diagramming
27. On the diagram below, sketch the path the light ray would take as it travels.

|
Answer and Explanation:
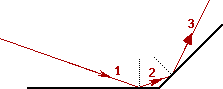
The path of the reflected ray at each boundary can be determined by sketching a normal at the point where the incident ray strikes the mirror surface. Once the normal is constructed the angle of incidence can be measured. For instance, the black dashed line at the location where ray 1 strikes the mirror is the normal line. The angle between ray 1 and the normal is approximately 62 degrees. The reflected ray should be drawn at the same angle from the normal - 62 degrees - but on the opposite side of the normal line. Ray 2 is thus drawn at a 62 degrees from the dashed normal line. Ray 2 is extended towards the second mirror. The process of measuring the angle of incidence and drawing the reflected ray (ray 3) is repeated.
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
28. Locate the images of the objects below and state which images can be seen by the eye.
|
Answer and Explanation:
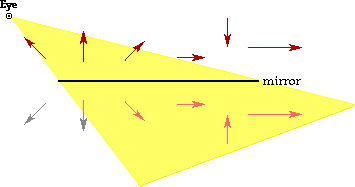
The images of the arrow objects can be found by using the principle that the image distance equals the object distance. The two extremities of the arrows can be used to find the two extremities of the image. The entire image will stretch from the image extremities.
The eye will be able to see any ray of light which bounces off the edges of the mirror or between the edges. The region shaded in yellow represents images within the visual field of this particular eye. When the yellow region is extended behind the mirror, it becomes obvious that the eye is unable to see the leftmost image and can see only a portion of the image located second from the left.
|
29. Locate all of the images formed by the mirror systems below.

|
Answer and Explanation:
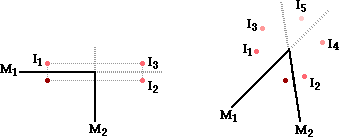
The images have been shown in pink above. The images and mirrors have been labeled to facilitate the discussion below.
For the right angle mirror, there are three images; two are primary images and the third is a secondary image. The primary images I1 and I2 are simple an image resulting from the reflection of light off of mirrors M1 and M2. When viewing these images, light from the object will reflect off one of these mirrors and travel towards the viewer's eye. The secondary image I3 is an image of an image. When viewing the secondary image, light must first reflect off one of the mirrors and then the second mirror before traveling towards the viewer's eye. Elaborate step-by-step directions for locating these images are found elsewhere; see the Physics Classroom tutorial page.
As the angle between mirrors is decreased as shown on the right, more images are formed. Two of these images are primary images - I1 and I2; the other images are images of images (I3 and I4) or images of images of images (I5). Image I1 is the image created by mirror M1 and image I2 is the image created by mirror M2. When viewing these images, light from the object will reflect off the respective mirror and travel towards the viewer's eye. I3 is the image of an image - the image of I2 as reflected across mirror M1. A quick measurement will reveal that the distance from I2 to mirror M1 is equal to the distance from image I3 to mirror M1. I4 is the image of an image - the image of I1 as reflected across mirror M2. A quick measurement will reveal that the distance from I1 to mirror M2 is equal to the distance from image I3 to mirror M2. When viewing the secondary images I3 and I4, light will reflect off both mirrors before traveling to the viewer's eye. Image I5 is the image of an image of an image. A quick measurement will review that the distance from I3 to mirror M2 is equal to the distance from I5 to mirror M2. Similarly, the distance from I4 to mirror M1 is equal to the distance from I5 to mirror M1. When viewing this image, light will reflect off one of the mirrors twice and the other mirror once before traveling to the viewer's eye.
|
|
30. Consider the following object in front of a plane mirror. Construct a ray diagram to show how light travels from the object (arrow) to the mirror and ultimately to the eye; then indicate the portion of the mirror needed in order for the eye to view the image.
|
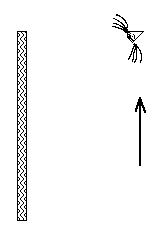
|
|
Answer and Explanation:
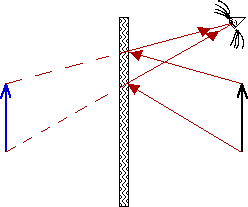
The image of the arrow object can be found by using the principle that the image distance equals the object distance. The two extremities of the arrow can be used to find the two extremities of the image. The entire image will stretch from the image extremities. This is shown in blue.
For the eye to see this image, it must sight along a line at the top of the image and the bottom of the image. As the eye sights along this line, a ray of light comes to the eye along this line of sight. This ray is the reflected ray. The light originates at the object and strikes the mirror at the location where the line of sight intersects the mirror. The light will automatically follow the law of reflection at the mirror (if the image is accurately located and the lines of sight are accurately drawn). The path of light is shown in red; note that dashed lines are used as extensions of the original line of sight extending behind the mirror.
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
31. For the right angle mirror below, draw in the secondary (or middle) image and show how light travels from the middle image to the eye.
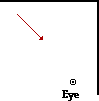
|
Answer and Explanation:
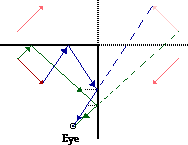
The primary and secondary images are drawn in a shade of pink. Once located, the ray diagram can be constructed. The principle that "to see an image, the eye must sight along a line at the image" is utilized. The set of green and blue lines represent the path of the light from the object to the eye. As the eye sights at the top of the middle image, light travels along the line of sight marked by the blue line and extended backwards to the top of the middle image. The light bouncing off the vertical mirror must first come from the horizontal mirror. The ray coming from the horizontal mirror to the vertical mirror is determined using the law of reflection. A normal line is drawn (in black) and the angle of reflection is measured; this angle is then used to determine the angle of incidence at the vertical mirror's surface. A ray is then traced backwards at this angle to the horizontal mirror. The light originated from the top of the object; thus, a ray is drawn from the top of the object to the horizontal mirror. These light rays for viewing the top of the image are drawn in blue in the diagram above. The process is repeated for viewing the bottom of the image; these rays are drawn in green.
Complete step-by-step instructions for right angle mirror ray diagrams are described elsewhere. See the Physics Classroom tutorial page.
|
[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | #10 | #11 | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 ]
32. Construct ray diagrams to show where the images of the following objects are located. Dray in the complete image and describe its characteristics (real or virtual, enlarged or reduced in size, inverted or upright). (NOTE: review the ray diagrams for all possible objects locations for each device.)
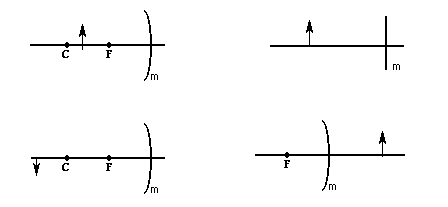
|
Answer:
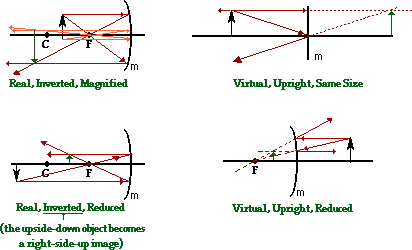
The images are drawn in green. The light rays are drawn in red or orange. The image descriptions are stated in green below each ray diagram. Elaborate step-by-step directions for constructing ray diagrams are provided on a separate page - see the links below. The plane mirror image is found by drawing any two (or more) rays and reflecting them according to the law of reflection. The reflected rays will always intersect at an image point.
|
Part C: Calculations and Explanations
33. Distinguish between diffuse and regular (specular) reflection in terms of both cause and result.
|
Answer:
Specular (or regular) reflection occurs when light reflects off a microscopically smooth surface. Light rays which are incident within a beam will reflect and remain in the beam.
Diffuse reflection occurs when light reflects off a microscopically rough surface. Light rays which are incident in a beam will diffuse (or scatter) and reflect in a variety of directions.
In each case the law of reflection holds; in diffuse reflection, the normals for each individual ray are not oriented in the same direction.
|
34. Write the formulae which show the relationship between image distance, object distance and focal length, and the magnification of an object/image.
|
Answer:
Please know this one:
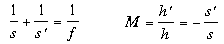
where s and h stand for object distance and object height,
and s' and h' stand for image distance and image height,
and f stands for focal length,
and M stands for magnification.
|
35. Fill in the blanks below for the problem-solving rules:
Focal lengths, object and image distances are ________ if they are on the reflective side of the mirror. Object and image heights are positive if they are ________ the principle axis.
|
Answer:
Focal lengths, object and image distances are positiveif they are on the reflective side of the mirror. Object and image heights are positive if they are belowthe principle axis.
The do and di values (s and s') and the hoand hi values (h and h') can have positive and negative values associated with them. In physics, a + or - in front of a quantity is always descriptive of some physical feature. In ray optics, a + distance (do, di or f) means in front of the mirror as the object; a negative distance means behind the mirror. A + height means above the principal axis and a - height means below the principal axis.
|
36. What is the focal length of a mirror which gives an image 3.00 meters away when an object is placed 150. cm from it?
|
Answer: 100. cm or 1.00 m
Use the mirror equation:
1/di + 1/do = 1/f
where di = +300. cm and do = +150. cm. (Note: since di > do, it must be a concave mirror and the image must be real.)
Substitute and solve for f.
1/(150. cm) + 1/(300. cm) = 1/f
1/f = 3.00/(300. cm) or 0.0100/cm
f = 100. cm = 1.00 m
|
37. Where is the image located when an object is placed 60.0 cm from a mirror which has a focal length of 20.0 cm?
|
Answer: 30.0 cm
Use the mirror equation:
1/di + 1/do = 1/f
where d0= +60.0 cm and f = +20.0 cm.
Substitute and solve for di.
1/(60.0 cm) + 1/di = 1/(20.0 cm)
1/di = 1/(20.0 cm) - 1/(60.0 cm) = 2/(60.0 cm)
di = 30.0 cm
|
38. How far would an object need to be placed from a mirror of focal length 10.0 cm if it is to produce an image which is 20.0 cm BEHIND the mirror?
|
Answer: 6.67 cm
Use the mirror equation:
1/di + 1/do = 1/f
where di= -20.0 cm and f = +10.0cm.
Substitute and solve for do.
1/do + 1/(-20.0 cm) = 1/(10.0 cm)
1/do = 1/(10.0 cm) - 1/(-20.0 cm) = 3.00/(20.0 cm) = 0.150/cm
do = 6.67 cm
|
39. A mirror with a focal length of -100. cm is used to form an image. An object is placed 50.0 cm in front of the mirror.
a. Where is the image located?
b. What type of mirror is being used in this problem?
|
Answer: -33.3 cm; convex mirror
Use the mirror equation:
1/di + 1/do= 1/f
where d0 = +50.0 cm and f = -100. cm. (Note: this is a convex mirror since the focal length is negative.)
Substitute and solve for di.
1/(50.0 cm) + 1/di = 1/(-100. cm)
1/di = 1/(-100. cm) - 1/(50.0 cm) = -3.00/(100. cm) or -0.03/cm
di = -33.3 cm
|
40. An object is placed 20 cm from a mirror of focal length 10.0 cm. The object is 5.0 cm tall. Where is the image located? How tall is the image?
|
Answer: di = 20.0 cm; hi = -5.0 cm
Use the mirror equation:
1/di + 1/do = 1/f
where d0 = +20.0 cm and f = 10.0 cm.
Substitute and solve for di.
1/(20.0 cm) + 1/di = 1/(10.0 cm)
1/di = 1/(10.0 cm) - 1/(20.0 cm) = 1/(20.0 cm) or 0.0500/cm
di = 20.0cm
Use the magnification ratio to solve for hi:
hi/ho = -di/do
where ho = 5.0 cm.
hi /(5.0 cm) = - (20.0 cm)/(20.0 cm)
hi = - [(20.0 cm)/(20.0 cm)]•(5.0 cm)
hi = -5.0 cm
|
41. A 20.0 cm tall object produces a 40.0 cm tall real image when placed in front of a curved mirror with a focal length of 50.0 cm. Determine the place that the object must be located to produce this image.
|
Answer: 75 ,.0cm
Given: ho = 20.0 cm, hi = -40.0 cm, f = 50.0 cm (Note: hi is negative since the image is real.)
Strategy: use the object and image height values with the magnification ratio in order to generate an expression for di in terms of do. Then substitute the expression into the mirror equation to solve for the object distance.
hi/ho = -di/do
(-40.0 cm)/(20.0 cm) = -di/do
-2.00 = = -di/do
di = 2.00*do
Now substitute this expression into the mirror equation.
1/do+ 1/(2.00*do) = 1/(50.0 cm)
2/(2.00*do) + 1/(2do) = 1/(50.0 cm)
3/(2.00*do) = 1/(50.0 cm)
2.00*do= 150. cm
do = 75.0 cm
|
42. A mirror is used to produce a virtual image that is 5.00 times bigger than the object. If the focal length of the mirror is 100. cm, where will the image be located?
|
Answer: -400. cm
Given: M = +5.00 , f = 100. cm (Note: M is positive since the image is virtual.)
Strategy: use the magnification value with the magnification ratio in order to generate an expression for do in terms of di. Then substitute the expression into the mirror equation to solve for the object distance.
M = hi/ho = -di/do
+5.00 = -di/do
do = = -di/5.00
Now substitute this expression into the mirror equation.
1/(-di/5.00)+ 1/(di) = 1/(100. cm)
-5.00/(di) + 1/(di) = 1/(100. cm)
-4.00/(di) = 1/(100. cm)
di = -400. cm
|
You Might Also Like ...
Users of The Review Session are often looking for learning resources that provide them with practice and review opportunities that include built-in feedback and instruction. If that is what you're looking for, then you might also like the following:
- The Calculator Pad
The Calculator Pad includes physics word problems organized by topic. Each problem is accompanied by a pop-up answer and an audio file that explains the details of how to approach and solve the problem. It's a perfect resource for those wishing to improve their problem-solving skills.
Visit: The Calculator Pad Home | Calculator Pad - Reflection and Mirrors
- Minds On Physics the App Series
Minds On Physics the App ("MOP the App") is a series of interactive questioning modules for the student that is serious about improving their conceptual understanding of physics. Each module of the series covers a different topic and is further broken down into sub-topics. A "MOP experience" will provide a learner with challenging questions, feedback, and question-specific help in the context of a game-like environment. It is available for phones, tablets, Chromebooks, and Macintosh computers. It's a perfect resource for those wishing to refine their conceptual reasoning abilities. Part 6 of the series includes topics in Reflection and Mirrors.
Visit: MOP the App Home || MOP the App - Part 6