Lesson 2: Gas Laws

Part a: Pressure and Temperature

The Experiment

Let’s begin our investigation of the gas laws by considering an experiment that is often performed in a Chemistry course. The experiment involves an air-filled flask that is equipped with a pressure sensor. The flask is sealed and rigid, preventing changes in the volume and the number of moles of gas. A thermometer is used to measure temperature. The flask is submerged in a beaker of chilled water. After adjusting to the temperature of the water, the pressure and temperature are measured. The process is repeated for several water samples of varying temperatures from just above 0°C up to 85°C. Sample data and the graph for such an experiment is shown below.

(Image Source: Vernier Science Education)
 


 
 

 

A Deeper Look at the Data

The data and graph show a clear pattern for the relationship between pressure and temperature. The relationship is linear and begs the mathematician in us to write an equation in y = m•x + b form. But not so fast. Let’s take a deeper look.
 
Let’s take the line on the graph and extend it leftward until it intersects with the x-axis. At what temperature does the pressure drop to 0 atm? The result is shown below.

 
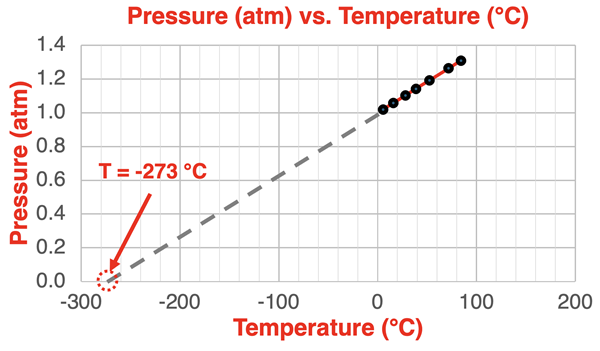
The temperature at which the pressure drops to 0 atm is approximately -273°C. Do you recognize that number? That is a temperature of 0 on the Kelvin temperature scale. That is generally referred to as absolute zero … the lowest temperature theoretically achievable. At this temperature, all particle motion ceases. At this temperature, particles will not collide with the container walls. There can be no pressure without such collisions. Particles are frozen in place and the pressure is 0.
 
There is one final adjustment that we will make to this graph. Let’s change the temperature scale to Kelvin and plot Pressure (atm) vs. Temperature (K).

 
Now we have a plot that is linear and has a y-intercept of 0. At this point we will give in to our inner mathematician and write an equation that fits the data. Using slope-intercept format (y = mx + b), we can assert that

Pressure (atm) = m • Temperature (K)

 where m represents the slope of the line. In the case of this gas sample, the slope is 0.00366 atm/K. Experiments of this nature have been repeated numerous times and the results confirm the conclusion that the pressure of a gas is directly proportional to its Kelvin temperature.
 
 

The Gay-Lussac Gas Law

Our simple experiment leads us to the first of many gas laws that we will be discussing in Lesson 2. It is often referred to as the Gay-Lussac law, in honor of French chemist Joseph Gay-Lussac. (There is some dispute as to whether the law was discovered by Gay-Lussac or Guillaume Amontons.) The law can be stated as follows:
 
For a sample of gas with a constant volume and number of moles, the pressure of the gas is directly proportional its Kelvin temperature.
 
This proportionality statement is often written in equation form as

P = k•T

 where P is the pressure, T is the Kelvin temperature, and k is a proportionality constant.

 
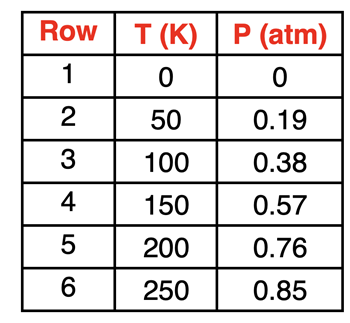
The idea of a directly proportional relationship indicates that the factor by which the Kelvin temperature is changed is the same factor by which the pressure changes. The sample data at the right illustrate this relationship. A doubling of the Kelvin temperature causes a doubling of the pressure. We see this pattern in Rows 2 and 3. The temperature is doubled from 50 K to 100 K and the pressure is doubled as a result, from 0.19 atm to 0.38 atm. Similarly, a tripling of the Kelvin temperature causes a tripling of the pressure. Once more, this is observed in Rows 2 and 4. The temperature is tripled from 50 K to 150 K and the pressure is tripled as a result, from 0.19 atm to 0.57 atm. Finally, a quadrupling of the Kelvin temperature causes a quadrupling of the pressure. We see this pattern in Rows 2 and 5. The temperature is quadrupled from 50 K to 200 K and the pressure is quadrupled from 0.19 atm to 0.76 atm.
 
 
 

The State Equation

As we have mentioned, a sample of gas is described by four variables - pressure, temperature, volume, and the number of moles. Gas samples often change the values of these variables. As we have seen on this page, the pressure and temperature change in direct proportion to one another. We might say that the gas sample changes from one state with a set of P-T-V-n conditions to another state with a different set of a set of P-T-V-n conditions. This is what we call a state change.
 
While the equation P = k•T communicates a useful proportionality, it is often more useful to express the relationship as a state equation. A state equation relates the variable values at one state to the variable values for another state after a change has occurred. The conversion of P = k•T to a state equation takes the following logical progression:

 
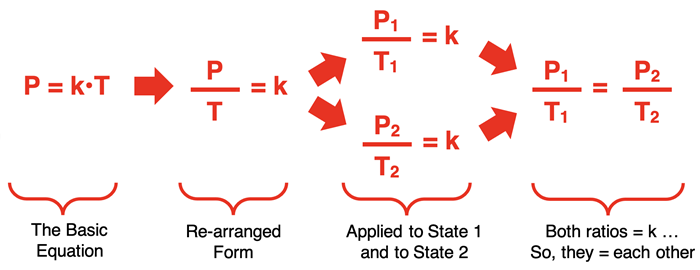
In the last step, a state equation is generated that relates the pressure and temperature in one state (P₁ and T₁) to the pressure and temperature of a second state (P₂ and T₂). This is one of the forms in which the Gay-Lussac law is commonly stated.
 

 
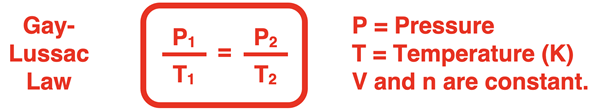
This state equation is often used in the solution of gas law problems. Here are two examples with accompanying solutions. Additional examples are provided in the Check Your Understanding section.
 

Example 1

Suppose a tennis ball contains 119 mL of gas and is pressurized to 32.0 psi at a temperature of 41.7°C. What is the internal pressure of the ball if the temperature drops to 4.2 °C? Assume a constant volume.
 

Solution

Example 2

The new helium gas cylinders have just arrived at the loading dock of a local party store. In the cool January air, the 20.0-L cylinders have a pressure of 68.6 atm. Once brought into the store, the cylinder’s temperatures increases to 27.1°C and the pressure adjusts to 76.1 atm. What is the outdoor temperature in °C?
 

Solution

Conclusion

The pressure-temperature relationship is the first of several gas laws presented in Lesson 2. We will take a very similar approach with each law, beginning with sample data and analysis and progressing to the use of a state equation that can be used to solve problems. Next up is the Volume and Temperature. But before you move onward, please check out our learning suggestions below.
 
 

Before You Leave

• Download our Study Card on Pressure and Temperature. Save it to a safe location and use it as a review tool. (Coming Soon)
• Try our Pressure and Temperature Concept Builder. It coordinates well with this lesson and provides exceptional practice.
• Problem Set GL3 in our Calculator Pad section provides six awesome practice problems. Visit Pressure and Temperature.
• The Check Your Understanding section below include questions with answers and explanations. It provides a great chance to self-assess your understanding.

Check Your Understanding

1. Identify two sets of two rows that illustrate that a doubling of the temperature will double the pressure. 
   
   Check Answer
   Answer: Rows 1 and 2 and Rows 2 and 4
   
   In both sets, the ratio of T values is 2:1 and the ratio of P values is 2:1.
   
    
   
    
2. Complete the sentence: Rows 1 and 5 illustrate that increasing the Kelvin temperature by a factor of ______ will cause the pressure to __________ (increase, decrease) by a factor of _____. 
   
   Check Answer
   Answer:
   Rows 1 and 5 illustrate that increasing the Kelvin temperature by a factor of 5 will cause the pressure to increase by a factor of 5.
   
    
   
    
3. Predict the value of the pressure when the Kelvin temperature is 360 K. 
   
   Check Answer
   Answer: 1.38 atm
   
   A temperature of 360 K is two times the temperature of Row 3. So the pressure will be two times the pressure of Row 3.
   
    
   
    
4. Predict the value of the pressure when the Kelvin temperature is 480 K. 
   
   Check Answer
   Answer: 1.84 atm
   
   A temperature of 480 K is two times the temperature of Row 4. So the pressure will be two times the pressure of Row 4.
   
    
   
    
5. Predict the value of the pressure when the Kelvin temperature is 150 K. 
   
   Check Answer
   Answer: 0.575
   
   A temperature of 150 K is midway between the Row 2 and Row 3 temperature. So the pressure should be midway between the Row 2 and Row 3 pressure.  Sincd the data are linear, you can simply interpolate. Take the difference in pressure between the two rows (0.23 atm). Halve this difference (.115 atm). Then add this value to the Row 2 pressure. (Excuse us for not following sig fig rules and posting the extra digit. Not doing so may have made it more confusing.)
    
   
    

1. Increase the pressure of the gas.
2. Decrease the pressure of the gas.