Lesson 4: Calculations in Chemistry
Part c: Significant Digits Revisited
Part 4a: The Factor Label Method
Part 4b: Unit Conversions
Part 4c: Significant Digits Revisited
Thinking Beyond the Rules
It is going to happen numerous times this year … to you and to other students in your class. Your teacher will do a calculation and report an answer like 4.7 grams. Being the conscientious type, you do the same calculation and get 4.6501438 grams. You are quite bothered. You didn’t get the same answer as your teacher. Your answer and her answer look quite different. Did you do it wrong? Is your answer close enough? Why does it look so different than your teacher’s answer? You raise your hand and you inquire and your teacher responds with I rounded to the second significant digit.
The response doesn’t sit well with you. It only raises more questions. Questions like … When do you round and when do you not round? Why did you round to the fourth significant digit yesterday and the second significant digit today? How much rounding can I do? Do I even have to round? What’s wrong with writing down my entire calculator panel? Why do you round up on Monday and round down on Tuesday? Why does this have to be so confusing?
These are all fair questions. But the fact is that there are rules for how your teacher handles and reports numbers. The rules can be a bit complicated and they do take time to grow on you. But more important than the rules for handling numbers is the logic behind the rules. When you understand the logic, the rules begin to make sense and many of the alternative ways of handling numbers seem unreasonable. Let’s talk about the logic behind the rules.
It’s About Precision
In Lesson 3, it was mentioned that every measurement contains three types of information. The three traits of a measurement are the …
- Numerical value
- Unit of measurement
- Degree of precision
The same can be said of any numerical quantity whether it be measured or calculated. It is the third trait – degree of precision - that is tied to the topic of significant digits. The number of significant digits in a reported value indicates how precisely we know that value.
Suppose that the same pencil is measured by two different people using two different measuring scales. Person A determines that the mass of a pencil is 7 grams. Person B, using a more expensive scale, determines the mass to be 7.281 grams. The two measurements agree with one another. One is
not more accurate than the other. But one is considerably more precise than the other. The more significant digits expressed in a numerical quantity, the more precisely that the quantity is reliably known.
Now suppose we attempt to determine Jeremy’s speed as he runs the 50-meter dash. Using a stopwatch, we determine that Jeremy runs 50.0 meters in 5.7 seconds. Using the speed formula (speed = distance/time), we calculate Jeremy’s speed and get …
Speed = (50.0 meter) / (5.7 second) = 8.7719298245 meter/second
(Do check our math; do this on your calculator.)
Jeremy’s speed is reported as 8.7719298245 meter/second.
Do you see anything illogical with this? The lack of logic does not lie in the accuracy of the calculated answer. The lack of logic lies i
n the reporting of the calculated answer. The reported answer - 8.7719298245 meter/second - is claiming a knowledge of Jeremy’s speed to a precision of 10 decimal places. There are 11
significant digits in the answer, yet the time measurement included only two
significant digits.
The logic behind the rules for reporting numerical information is that a calculated answer cannot be more precise than the numbers used to calculate it. You cannot know distance and time to two or three significant digits and then perform a calculation and know the answer to 11 significant digits. Calculations can provide you with knowledge of another quantity – in this case, speed – but calculations cannot increase the precision to which you know that quantity. It is not logical to measure time with a $5 stopwatch and then to calculate the speed to a level of precision that is only capable from the use of a multi-thousand-dollar time clock.
Your Calculator has a Problem
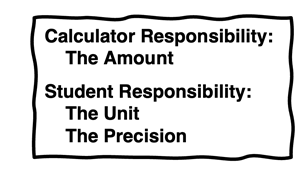
Calculators are handy devices. But sorry; they do not understand the limits on how precisely a calculated quantity is known. Calculators simply report a collection of numbers. It is your job to make meaning of the numbers. Part of meaning-making is to determine how many of the digits on the LCD panel have significance. We will be discussing how to determine this value soon. But for now, you need to understand that there are three traits to every numerical quantity and your calculator can only manage one of them. You must manage the other two. The calculator can determine the amount. But the Chemistry student must determine the unit and the degree of precision to which the amount is reliably known. You must acquire the amount from the calculator and report it with a unit and the proper number of
significant digits.
Rule for Addition and Subtraction
The logic emphasized above is that a calculation cannot increase (or decrease) the level of precision to which you understand the world. The rule for
significant digits on calculated answers reflects this logic. When adding or subtracting two or more numbers, the number of decimal places in the result is equal to the number of decimal places in the quantity with the least certainty (i.e., least number of decimal places).
Example 1: A = 1.2 and B = 1.328947. What is A + B?
Answer: A + B = 2.5
Explanation: Adding 1.2 to 1.328947 on your calculator leads to an amount of 2.528947. But the certainty to which you know the sum is limited by the fact that you know A to the first decimal place. As such, the sum can only be known to the first decimal place.
Example 2: A = 32 and B = 1.416. What is A + B?
Answer: A + B = 33
Explanation: Adding 32 to 1.416 on your calculator leads to an amount of 33.416. But the certainty to which you know the sum is limited by the fact that you know A to one’s place. As such, the sum can only be known to the one’s place.
Example 3: A = 1.328947 and B = 1.2. What is A - B?
Answer: A - B = 0.1
Explanation: Subtracting 1.2 from 1.328947 on your calculator leads to an amount of 0.128947. But the certainty to which you know the sum is limited by the fact that you know B to the first decimal place. As such, the A-B can only be known to the first decimal place.
Rule for Multiplying and Dividing
The addition and subtraction rule is based upon the number of decimal places. The rule for multiplication and division is a bit different. It is based upon the number of
significant digits. When multiplying or dividing two or more numbers, the number of significant digits on the result is the same as the quantity with the least significant digits.
Example 1: A = 1.2 and B = 1.328947. What is A • B?
Answer: A • B = 1.6
Explanation: Multiplying 1.2 by 1.328947 on your calculator leads to an amount of 1.5947364. But the certainty to which you know the product is limited by the fact that you know A is expressed with two significant digits. As such, the product is reported with two significant digits. Note that the 1.5947364 value is rounded upward to 1.6. We will discuss rounding rules later on this page.
Example 2: A = 12 and B = 3.6. What is A • B?
Answer: A • B = 43
Explanation: Multiplying 12 by 3.6 on your calculator leads to an amount of 43.2. But the certainty to which you know the product is limited by the fact that both A and B are expressed with two significant digits. As such, the product is reported with two significant digits.
Example 3: A = 7 and B = 3. What is A ÷ B?
Answer: A • B = 2
Explanation: Dividing 7 by 3 on your calculator leads to an amount of 2.3333333333. But the certainty to which you know the product is limited by the fact that both A and B are expressed with 1 significant digit. As such, the product is reported with one significant digit.
Many students will be bothered by Example 3 because they learned in math that 6÷3 is 2; so surely 7÷3 cannot also be equal to 2. Always remember this discussion is about precision and a calculation cannot increase the precision or certainty to which we know the world.
Exact Numbers
There are numbers that we refer to as
exact numbers. For instance, we might state that there are 25 people in the Chemistry classroom. The 25 is an exact number. Exact numbers can be treated as having an infinite number of decimal places and infinite number of significant digits. When using exact numbers in calculations, they do not limit the amount or precision to which we know the calculated result.
Examples of exact numbers include:
- Counted Values; like the number of people in a room
- Metric Conversion Factors; the 1, 10, 1000, etc. used to convert metric units
- Constants found in Formulas; like the number 2 in diameter = 2•radius
Rounding Off Rules
In Chemistry, we do not
truncate numbers. Rather we
round numbers upward or downward. Truncating involves eliminating digits from the end of the number; the digits are cut off at a certain place. Rounding is a bit more sophisticated. There is typically a rule that is used that determines whether or not the last digit of the reported value is rounded upward by 1. Let’s first look at several rounding examples. See if you can determine the rule. Then we will state the rule and the method that we will use in our
Chemistry Tutorial.
- Round 3.42 to the first decimal place → 3.4
- Round 3.48 to the first decimal place → 3.5
- Round 3.45 to the first decimal place → 3.5
- Round 3.42964 to the first decimal place → 3.4
- Round 3.449999 to the first decimal place → 3.4
- Round 3.450000 to the first decimal place → 3.5
- Round 26.49 to the one’s place → 26
- Round 26.5 to the one’s place → 27
- Round 26.5 to the ten’s place → 30
- Round 24.9 to the ten’s place → 20
Suppose you have a calculated number and you must report it to a particular decimal place or a particular number of significant digits. How would you go about doing that? How do you know to round up or round down? The 3-step method is demonstrated in the three examples of the graphic below.
Multi-step Operations and Rounding
Many Chemistry problems involve multiple calculations. The result from the first calculation is used in the second calculation. And the result from the second calculation is used in the third calculation. And so forth. When conducting multiple-step calculations that lead towards a
final answer, it is important to avoid preliminary rounding. A rounded version of the first calculation result should not be used in the second calculation. Use all the digits you have and round after the final step. If you need to report the results of the individual steps, you can report it to the proper number of significant digits. But use the unrounded result in any calculation that follows. The diagram below shows what happens when results from preliminary steps of a multi-step calculation are rounded. The final result is 3% different as a result of the early rounding. If you use an automated problem delivery and grading system (like our Calculator Pad … and many others), you are typically allowed a 2% error allowance. Early rounding would result in being outside of this range and result in an incorrect answer.
Pep Talk
Learning how to appropriately handle numerical information will take some time. It begins with the acceptance that there are three traits to all numerical quantities – amount, unit*, and precision level. Don’t let difficulty in learning this third trait – precision level - interfere with other concepts you need to learn in Chemistry class. Be patient with significant digits and precision. Give this lesson some time because it will take some time to
grow on you. Treat this lesson as important and do your due diligence to learn it as you continue through the course. But don’t hyper-fixate upon it at every turn or get frustrated with Chemistry when it is difficult. It’s a small, small part of Chemistry so don’t treat it as if were the
be all and end all. And always use logic since the lesson is based in logic.
Check Your Understanding
Use the following questions to assess your understanding. Tap the Check Answer buttons when ready.
1. A student lab group is attempting to determine the volume of the room. They measure length, width, and height and calculate the volume. Their work is shown below.
What have they done wrong? Explain. Then correct their final answer.
2. Use the rounding methods discussed on this page to properly report the following calculator panel numbers.
- Report 562.849721 to the first decimal place.
- Report 5.59043628 to the third decimal place.
- Report 0.012928445 to the fourth decimal place.
- Report 0.012928445 to two significant digits.
- Report 103.720995 to three significant digits.
- Report 103.720995 to two significant digits.
- Report 4.99521883 to three significant digits.
3. Conduct the following operations and report the result to the proper precision.
- 425 + 33.2
- 425 – 33.2
- 2.656 -1.2
- 100.0 + 2.45
- 3.85 x 42.1
- 0.00385 x 42100
- 2 x 14.3
- 2.0 x 14.3
- 2.00 x 14.3
- 1.248 + 2.1 * 5.0
- (1.248 + 2.1) * 5.0
- (1.248 + 2.1) / 5.0
- (2.1 - 1.248) / 5.0