Waves are everywhere. Whether we recognize it or not, we encounter waves on a daily basis. Sound waves, visible light waves, radio waves, microwaves, water waves, sine waves, cosine waves, stadium waves, earthquake waves, waves on a string, and slinky waves and are just a few of the examples of our daily encounters with waves. In addition to waves, there are a variety of phenomena in our physical world that resemble waves so closely that we can describe such phenomenon as being wavelike. The motion of a pendulum, the motion of a mass suspended by a spring, the motion of a child on a swing, and the "Hello, Good Morning!" wave of the hand can be thought of as wavelike phenomena. Waves (and wavelike phenomena) are everywhere!

We study the physics of waves because it provides a rich glimpse into the physical world that we seek to understand and describe as students of physics. Before beginning a formal discussion of the nature of waves, it is often useful to ponder the various encounters and exposures that we have of waves. Where do we see waves or examples of wavelike motion? What experiences do we already have that will help us in understanding the physics of waves?

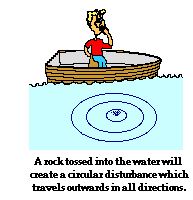For many people, the first thought concerning waves conjures up a picture of a wave moving across the surface of an ocean, lake, pond or other body of water. The waves are created by some form of a disturbance, such as a rock thrown into the water, a duck shaking its tail in the water or a boat moving through the water. The water wave has a crest and a trough and travels from one location to another. One crest is often followed by a second crest that is often followed by a third crest. Every crest is separated by a trough to create an alternating pattern of crests and troughs. A duck or gull at rest on the surface of the water is observed to bob up-and-down at rather regular time intervals as the wave passes by. The waves may appear to be plane waves that travel together as a front in a straight-line direction, perhaps towards a sandy shore. Or the waves may be circular waves that originate from the point where the disturbances occur; such circular waves travel across the surface of the water in all directions. These mental pictures of water waves are useful for understanding the nature of a wave and will be revisited later when we begin our formal discussion of the topic.

The thought of waves often brings to mind a recent encounter at the baseball or football stadium when the crowd enthusiastically engaged in doing the wave. When performed with reasonably good timing, a noticeable ripple is produced that travels around the circular stadium or back and forth across a section of bleachers. The observable ripple results when a group of enthusiastic fans rise up from their seats, swing their arms up high, and then sit back down. Beginning in Section 1, the first row of fans abruptly rise up to begin the wave; as they sit back down, row 2 begins its motion; as row 2 sits back down, row 3 begins its motion. The process continues, as each consecutive row becomes involved by a momentary standing up and sitting back down. The wave is passed from row to row as each individual member of the row becomes temporarily displaced out of his or her seat, only to return to it as the wave passes by. This mental picture of a stadium wave will also provide a useful context for the discussion of the physics of wave motion.
 

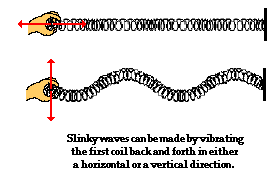Another picture of waves involves the movement of a slinky or similar set of coils. If a slinky is stretched out from end to end, a wave can be introduced into the slinky by either vibrating the first coil up and down vertically or back and forth horizontally. A wave will subsequently be seen traveling from one end of the slinky to the other. As the wave moves along the slinky, each individual coil is seen to move out of place and then return to its original position. The coils always move in the same direction that the first coil was vibrated. A continued vibration of the first coil results in a continued back and forth motion of the other coils. If looked at closely, one notices that the wave does not stop when it reaches the end of the slinky; rather it seems to bounce off the end and head back from where it started. A slinky wave provides an excellent mental picture of a wave and will be used in discussions and demonstrations throughout this unit.

We likely have memories from childhood of holding a long jump rope with a friend and vibrating an end up and down. The up and down vibration of the end of the rope created a disturbance of the rope that subsequently moved towards the other end. Upon reaching the opposite end, the disturbance often bounced back to return to the end we were holding. A single disturbance could be created by the single vibration of one end of the rope. On the other hand, a repeated disturbance would result in a repeated and regular vibration of the rope. The shape of the pattern formed in the rope was influenced by the frequency at which we vibrated it. If we vibrated the rope rapidly, then a short wave was created. And if we vibrated the rope less frequently (not as often), a long wave was created. While we were likely unaware of it as children, we were entering the world of the physics of waves as we contentedly played with the rope.

Then there is the "Hello, Good Morning!" wave. Whether encountered in the driveway as you begin your trip to school, on the street on the way to school, in the parking lot upon arrival to school, or in the hallway on the way to your first class, the "Hello, Good Morning!" wave provides a simple (yet excellent) example of physics in action. The simple back and forth motion of the hand is called a wave. When Mom commands us to "wave to Mr. Smith," she is telling us to raise our hand and to temporarily or even repeatedly vibrate it back and forth. The hand is raised, moved to the left, and then back to the far right and finally returns to its original position. Energy is put into the hand and the hand begins its back-and-forth vibrational motion. And we call the process of doing it "waving." Soon we will see how this simple act is representative of the nature of a physical wave.

We also encountered waves in Math class in the form of the sine and cosine function. We often plotted y = B•sine(A•x) on our calculator or by hand and observed that its graphical shape resembled the characteristic shape of a wave. There was a crest and a trough and a repeating pattern. If we changed the constant A in the equation, we noticed that we could change the length of the repeating pattern. And if we changed B in the equation, we noticed that we changed the height of the pattern. In math class, we encountered the underlying mathematical functions that describe the physical nature of waves.

Finally, we are familiar with microwaves and visible light waves. While we have never seen them, we believe that they exist because we have witnessed how they carry energy from one location to another. And similarly, we are familiar with radio waves and sound waves. Like microwaves, we have never seen them. Yet we believe they exist because we have witnessed the signals that they carry from one location to another and we have even learned how to tune into those signals through use of our ears or a tuner on a television or radio. Waves, as we will learn, carry energy from one location to another. And if the frequency of those waves can be changed, then we can also carry a complex signal that is capable of transmitting an idea or thought from one location to another. Perhaps this is one of the most important aspects of waves and will become a focus of our study in later units.

Waves are everywhere in nature. Our understanding of the physical world is not complete until we understand the nature, properties and behaviors of waves. The goal of this unit is to develop mental models of waves and ultimately apply those models to an understanding of the two most common types of waves - sound waves and light waves.