Electric Circuits Review

The Physics Classroom » The Review Session » Electric Circuits » Answers

Electric Circuits Review

Navigate to:

Review Session Home - Topic Listing

Electric Circuits - Home || Printable Version || Questions with Links

Answers to Questions: All || #1-7 || #8-51 || #52-59 || #60-72

[ #1 | #2 | #3 | #4 | #5 | #6 | #7 | #8 | #9 | | | #12 | #13 | #14 | #15 | #16 | #17 | #18 | #19 | #20 | #21 | #22 | #23 | #24 | #25 | #26 | #27 | #28 | #29 | #30 | #31 | #32 | #33 | #34 | #35 | #36 | #37 | #38 | #39 | #40 | #41 | #42 | #43 | #44 | #45 | #46 | #47 | #48 | #49 | #50 | #51 | #52 | #53 | #54 | #55 | #56 | #57 | #58 | #59 | #60 | #61 | #62 | #63 | #64 | #65 | #66 | #67 | #68 | #69 | #70 | #71 | #72 ]

Part A: Multiple-Multiple Choice

1. Which of the following statements are true about an electric circuit? List all that apply.

Electrons are the mobile charge carriers in an electric circuit.
The path of charge flow from the + to the - terminal of the circuit can consist of nonconductive material.
In an electric circuit of an automobile, the 12-Volt car battery is sometimes referred to as the internal circuit because it is located inside of the hood of the car.
Charge is supplied with energy in the internal circuit and the energy is transformed into other forms in the external circuit.
Charge is consumed as it moves through the energy-transforming devices of the external circuit. For instance, the amount of charge entering a light bulb in a second is less than that which exits the light bulb in a second.
A battery is used to power a flashlight circuit. When the battery no longer works, it is because it has run out of charge.
The location on an electric circuit where the charge possesses the greatest amount of electric potential energy is the + terminal of the battery.

Answer: ADG

a. TRUE - Physicists often speak of conventional current as the direction that positive charge moves through a circuit. This is based on the convention that the direction of the electric field is the direction that a + test charge would be accelerated. In reality, the carrier of electric charge in an electric circuit is the electron.

b. FALSE - For charge to flow through an external circuit, the pathway must consist of conductive materials. The presence of a nonconductor would inhibit charge flow and low the current to zero.

c. FALSE - The internal circuit is the portion of the circuit inside the battery, located between the - terminal and the + terminal. Whether the battery is inside the hood or not has nothing to do with it. If you were to place your battery on top of your hood, it would still be the internal circuit.

d. TRUE - The battery is the internal circuit; it is the place where charge acquires its energy. The external circuit consists of wires, light bulbs, resistors, etc. In the external circuit, charge has its electrical energy transformed into other forms as it passes through the elements of the external circuit.

e. FALSE - Charge is neither created nor destroyed, and as such, the amount of charge which enters a light bulb in a second is equal to the amount of charge which exits the light bulb in a second. This quantity (charge per second) is the current and it is everywhere the same in a circuit.

f. FALSE - Batteries produce energy through a exothermic chemical reaction. When a battery no longer works, the ability of its chemical reaction to produce products and energy has become limited by the depletion of reaction and the build up of products. It has not run out of charge; it has run of out chemical reactants.

g. TRUE - Know this one! The + terminal is the location of high energy in an electric circuit.

Useful Web Links

What is an Electric Circuit? || Requirements of a Circuit

2. Which of the following statements are true about an electric potential or electric potential difference? List all that apply.

Electric potential can be expressed in units of volts.
Electric potential can also be expressed in units of Joules.
The electric potential of a charge at a given location provides a measure of the rate at which charge flows past that point.
Work must be done on a + charge to move it against (i.e., in the opposite direction of) an electric field.
As a + charge moves in the same direction as an electric field, it gains electric potential.
The electric potential difference between two points is simply the difference in potential energy possessed by charge between those two points.
If a unit of charge has a high electric potential at any given location, then that charge possesses a large amount of electric potential energy at that location.
The + terminal of a battery is a location with a higher electric potential than the - terminal of a battery.
Charge flowing through a battery will experience a gain in electric potential.
A 6-Volt battery would provide 12 Joules of energy to 2 Coulombs of charge as it is moved from the - to the + terminal.

Answer: ADGHIJ

a. TRUE - Electric potential, also known as voltage, is measured in units of volts.

b. FALSE - The electric potential at a given location in a circuit is defined the electric potential energy per charge at the location. Its units would be energy units per charge units - a Joule per Coulomb (not simply a Joule).

c. FALSE - The rate at which charge flows past a point is the current. Electric potential is the potential energy per charge at a given location.

d. TRUE - By convention, an electric field is the direction which a positive test charge would be pushed or pulled. If a positive charge is moved against an electric field, then work must be done upon the charge to overcome the opposing effect of the field.

e. FALSE - A positive charge moving in the direction of an electric field is moving without any external help. Such a charge is analogous to a bicycle moving downhill; the bicyclist would not have to pedal. In such instances, there is a loss in electric potential energy, and thus a loss in electric potential.

f. FALSE - Close, but not correct. The difference in electric potential between any two points is simply the different in electric potential energy per charge between those two points. Using a gravitational analogy, gravitational potential is the difference in gravitational potential energy per mass between any two points. Gravitational potential energy is dependent upon the amount of mass present at a location (m•g•h); the more mass present at a location , the more gravitational potential energy. In contrast, gravitational potential reduces the importance of mass by defining itself as the potential energy per kilogram of mass (PE / m). By so doing, gravitational potential is now only location dependent - depending only upon the value of g at a given location and the height above some zero reference level. In the same manner, electric potential energy at a given location is charge-dependent. The more charge present at a location, the more repulsive (or attractive) effects and the greater the potential energy. But electric potential makes the amount of charge unimportant since it is the electric potential energy per charge. Now electric potential is location dependent - dependent for instance on the location in a circuit and not upon the amount of charge flowing past that location in a second.

g. TRUE - Electric potential is the potential energy per charge at a given location. The more electric potential which a unit of charge has at a given location, the more electric potential energy that the charge has.

h. TRUE - In the battery, the positive terminal is the location of high energy and high potential. The negative terminal is the location of low energy and low potential.

i. TRUE - In the battery, a charge is moved from a location of low energy to a location of high energy. The charge would gain electric potential energy and as such, it would also gain electric potential.

j. TRUE - A 6-Volt battery supplies every Coulomb of charge with 6 Joules of energy. The energy per charge ratio is 6 Joules per Coulomb. Thus, 2 Coulombs of charge would gain 12 Joules of energy.

Useful Web Links

Electric Field and the Movement of Charge || Electric Potential || Electric Potential Difference

3. Which of the following statements are true about electric current? List all that apply.

Electric current is measured in units of Amperes.
Electric current and drift speed are synonymous terms.
Electric current is defined as the number of Coulombs of charge which move past a point on a circuit.
Electric current is equal to the number of Coulombs of charge which move past a point on a circuit per unit of time.
Electric current provides a measure of how fast charge moves between two points on a circuit.
The electric current diminishes in value as charge progresses to locations further and further from the + terminal of the battery.
The electric current in a circuit will increase as the electric potential impressed across a circuit is increased.
The electric current in a circuit will triple in value as the electric potential impressed across a circuit is increased by a factor of three.
Suppose a miniature light bulb is connected to a battery in a circuit. A light bulb with a greater resistance will have a greater current.
Wider conducting wires are capable of carrying larger currents.
If one starts getting technical about the meaning of electric current, one could argue that electric current is not something that flows past a point on a circuit, but rather something that exists to a given measure at a point on a circuit.

Answer: ADGHJK

a. TRUE - Electric current is the rate at which charge flows past a point on a circuit. It is measured in Coulombs per second, also known as an Ampere or an "Amp."

b. FALSE - No! Current refers to how many Coulombs of charge pass a cross-sectional area in a wire in a second of time. Drift speed refers to the distance that a mobile charge carrier travels in a unit of time. One quantity - current - has to do with how many? and the other quantity - drift speed - has to do with how far?

c. FALSE - Current is a rate quantity much like velocity or power is a rate quantity. There is a per time basis to it. Current is defined as the Coulombs of charge which pass a point on a circuit per second of time.

d. TRUE - Yes! This is the definition of current - a definition which ought to be committed to memory.

e. FALSE - See the explanation to part b above.

f. FALSE - Charge is marching around a circuit at the same rate everywhere within the circuit. The number of Coulombs which exit the battery at the + terminal in a second is equal to the number of Coulombs which enter the battery at the - terminal in a second. Charge is not created; charge is not destroyed; and charge does not accumulate at a location in a circuit. Charge simply marches together around the loop of a circuit. The quantity which is lost and gained is energy. Work is done on the charge as it passes through the battery, thus pumping the charge up to a high energy. And the charge subsequently does work upon the elements of the external circuit, transforming its electric potential energy to other useful forms (light, sound, mechanical, thermal, etc.).

g. TRUE - A higher electric potential impressed across the two terminals of a battery will cause the rate of charge flow (i.e., current) to increase. This is consistent with the V = I•R equation.

h. TRUE - Consistent with V = I•R, one could say that the current is directly proportional to the voltage. A tripling of the voltage (while R is held constant) would cause a tripling of the current.

i. FALSE - A light bulb with a greater resistance will offer more hindrance to the flow of charge through it and as such lead to less current. Since I = V / R, the quantities current and resistance are inversely proportional.

j. TRUE - A wider wire has a greater cross-sectional area and thus offers less resistance. As such, wider wires allow a greater current or flow rate.

k. TRUE - Technically speaking, current does not flow or move. Current is a quantity which is measured at a location and is based upon the rate at which charge flows. Teachers and textbooks often get sloppy with their language and say that the current flows. In reality, it is the charge that does the moving; the current is simply measured at a location to reveal the rate at which charge flows past that location.

Useful Web Links

Electric Current || Common Misconceptions Regarding Electric Circuits || Journey of a Typical Electron

4. Which of the following statements are true about an electric resistance? List all that apply.

The resistance of an electric circuit is a measure of the overall amount of hindrance to the flow of charge through the circuit.
A large resistance value indicates that the charge is encountering a relatively large amount of difficulty in moving through the circuit.
The unit of resistance is the ampere.
A 5-ohm resistor would allow a current of 0.5 Amperes when 2.5 Volts is impressed across it.
A 10-ohm resistor would allow a current of 2 Amperes when 5 Volts is impressed across it.
The resistance of a conducting wire will increase as the length of the wire is increased.
The resistance of a conducting wire will increase as the cross-sectional area of the wire is increased.
Increasing the resistance of an electric circuit will cause the current in the circuit to increase.
A threefold increase in the resistance of an electric circuit will result in a threefold decrease in the electric current.
A miniature light bulb with a specific resistance is connected to a 1.5-Volt battery to form a circuit. If it were connected to a 6-Volt battery instead, its resistance would increase by a factor of 4.

Answer: ABDFI

a. TRUE - Yes! Remember this as the definition of resistance.

b. TRUE - Resistance refers to the amount of hindrance (resistance) to the flow of charge. The more hindrance which charge encounters, the more resistance.

c. FALSE - The unit of resistance is the ohm. Ampere or Amps is the unit of current.

d. TRUE - The resistance of a resistor is the V/I ratio at that location. A 5-ohm resistor would have a 5:1 ratio of voltage to current. This is a true statement since (2.5 Volt) / (0.5 A) is equal to 5.0 Volt/Amp.

e. FALSE - The resistance of a resistor is the V/I ratio at that location. A 10-ohm resistor would have a 10:1 ratio of voltage to current. This is a false statement since (5 Volt) / (2 A) is not equal to 10 Volt/Amp.

f. TRUE - The resistance of a wire is mathematically related to the resistively of the material, the length of the wire and the cross-sectional area of the wire. Longer wires have a greater resistance.

g. FALSE - The resistance of a wire is mathematically related to the resistively of the material, the length of the wire and the cross-sectional area of the wire. Wider wires have a lower resistance.

h. FALSE - More resistance would cause less current.

i. TRUE - In accordance with the equation I = V / R, current is inversely proportional to the resistance. Tripling the resistance will cause the current to decrease by a factor of three.

j. FALSE - The resistance of a light bulb is an intrinsic property which depends upon the material and dimensions (length and diameter) of the light bulb filament. If the same light bulb is connected to a battery of greater potential, only the current at the bulb location will be modified.

Useful Web Links

Journey of a Typical Electron || Resistance || Ohm's Law

5. Which of the following statements are true about electric power and electric potential energy? List all that apply.

Power is the amount of energy used by an electrical appliance.
Power is the rate at which energy is used by an electric circuit.
A kiloWatt•hour is a unit of electric power.
A Joule/second is a unit of power.
A 60-Watt light bulb would consume 30 Joules of electrical energy in 2 seconds.
A 120-Watt light bulb would consume 1200 Joules of electrical energy in 6 seconds.
A charge moving through an electric circuit would gain electric potential energy in the internal circuit and lose electric potential energy in the external circuit.
Charge has the greatest amount of electric potential energy at the - terminal of the battery.
If a 60-Watt light bulb is placed in a 120-Volt circuit, then the current in the light bulb is 2 Amps.
Consider two light bulbs with different power ratings. A 60-Watt light bulb has a greater resistance than a 100-Watt light bulb.

Answer: BDGJ

a. FALSE - Power is a rate quantity; it is the rate at which energy is used by an electrical appliance.

b. TRUE - Yes! Know this definition.

c. FALSE - A kiloWatt is a unit of power. An hour is a unit of time. Power is energy per time. So when power is multiplied by time, the result is energy. A kiloWatt•hour is a unit of energy.

d. TRUE - Power is the rate at which energy is used by an electrical appliance. As such, power is measure in energy per time units - Joules per second.

e. FALSE - Power is the energy per time ratio. A 60-Watt bulb would consume 60 Joules of energy in 1 second, 120 Joules of energy in 2 seconds, and 30 Joules of energy in 0.5 seconds.

f. FALSE - Power is the energy per time ratio. A 120-Watt bulb would consume 120 Joules of energy in 1 second, 720 Joules of energy in 6 seconds, and 1200 Joules of energy in 10 seconds.

g. TRUE - This is how an electric circuit work. The internal circuit supplies electrical energy to the charge and the electrical energy is converted to other forms of energy in the external circuit.

h. FALSE - The - terminal is the location of lowest energy in an electric circuit. the location of highest energy is the + terminal.

i. FALSE - Power is related to voltage and current by the equation P = I•V. So a 60-Watt bulb would allow a current of 0.5 Amps if wired into a 120-Volt circuit.

j. TRUE - A 60-Watt light bulb and a 100-Watt light bulb have different resistance values, which in turn effects the current at the light bulb location. The bulb with the greatest resistance has the lower current. So which bulb - high power or low power - has the greatest resistance? One must consider the expression for power which includes both the current (I) and the resistance (R) since the choice of bulb will effect both variables. The equation P = I²•R is of utmost importance here. In the equation, the I is of double the importance since it is the square term in the equation. So the bulb with the greatest I is the bulb with the greatest power. The 100-Watt bulb has the greatest current; and therefore it must have the lowest resistance. The 60-Watt bulb has the greatest resistance.

Useful Web Links

Power: Putting Charges to Work || Power Revisited

6. Which of the following statements are true about a series circuit? List all that apply.

Series circuits are characterized by the fact that there is a single pathway by which charge can travel from the + terminal to the - terminal.
During any single loop of charge around a series circuit, the charge will pass through each resistor in the circuit.
The overall resistance of a circuit increases as more and more resistors are placed in series in the circuit.
The total current in a circuit increases as more and more resistors are placed in series in the circuit.
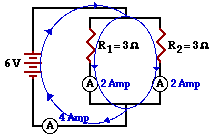
Suppose that three identical resistors with a resistance of 3-ohms are placed in series with a 12-Volt battery. The current in the circuit is 4 Amps.
Suppose that three identical resistors with a resistance of 3-ohms are placed in series with a 12-Volt battery. The electric potential difference across each resistor is 12 Volts.
Suppose that two identical resistors are placed in series with a 12-Volt battery. The current in the circuit is 6 amps. The resistance of each resistor is 4 ohms.

Answer: ABC

a. TRUE - Series circuits are circuits which allow a single pathway for charge to follow; there is no no branching locations in a series circuit.

b. TRUE - Since there are no branching points in a series circuit, charge is never left with a choice of passing through either Resistor A or Resistor B. It must pass through both resistors in consecutive fashion before returning to the - terminal of the battery.

c. TRUE - Adding more resistors in series would mark the overall resistance of the circuit increase since each charge making a loop around the circuit will encounter each of these resistors.

d. FALSE - If adding more resistors makes the overall resistance of the circuit increase, then it will make the overall current in the circuit decrease. For a constant battery voltage, current and resistance are inversely proportional.

e. FALSE - Three resistors wit a resistance of 3 Ohms placed in series is equivalent to a single resistor with a resistance of 9 Ohms. The current through the circuit would be equal to the battery voltage divided by the overall or equivalent resistance. I = (V_Tot) / (R_Tot) = (12 Volts) / (9 Ohms) = 1.33 Amps.

f. FALSE - In a series circuit, the voltage gained by the charge in passing through the battery - in this case, 12 Volts - is equal to the overall or cumulative voltage drop of the charge as it passes through the resistors. So the sum of the voltage drops in each of the three individual resistors must be 12 Volts. In the case of three resistors of equal resistance, the charge will lose 4 Volts in each resistors, thus accumulating a total voltage drop of 12 Volts.

g. FALSE - The total or equivalent resistance of these two series resistors is simply the ratio of the battery voltage to the overall current. That is, R_Tot = (V_Tot) / (I_Tot) = (12 Volts) / (6 Amps) = 2 Ohms. For resistors in series, the overall or total resistance is the sum of the individual resistances. Thus, each resistor must have a resistance of 1 Ohm.

Useful Web Links

Two Types of Connections || Series Circuits

7. Which of the following statements are true about a parallel circuit? List all that apply.

Parallel circuits are characterized by the fact that there are multiple pathways by which charge can travel from the + terminal to the - terminal.
During any single loop of charge around a parallel circuit, the charge will pass through each resistor in the circuit.
The overall resistance of a circuit increases as more and more resistors are placed in parallel in the circuit.
The total current in a circuit increases as more and more resistors are placed in parallel in the circuit.
Suppose that three identical resistors with a resistance of 3-ohms are placed in parallel with a 12-Volt battery. The current in the circuit is 1 Amp.
Suppose that three identical resistors with a resistance of 3-ohms are placed in parallel with a 12-Volt battery. The electric potential difference across each resistor is 12 Volts.
Suppose that two identical resistors are placed in parallel with a 12-Volt battery. The overall current in the circuit is 6 amps. The resistance of each resistor is 4 ohms.

Answer: ADFG

a. TRUE - This is the definition of a parallel circuit. A parallel circuit contains a branching location. When a charge reaches the branching location, it may choose between a pathway which includes any one of at least two resistors.

b. FALSE - This is a characteristic of a series circuit. In a parallel circuit, charge makes a either-or choice at the junction or branching locations, choosing to pass through either Resistor A or Resistor B. It does not pass through both of them in consecutive fashion, but only through one or the other resistor.

c. FALSE - Increasing the number of resistors in parallel branches will have the effect of decreasing the overall resistance of the circuit. At first this seems rather counterintuitive. But consider that the new resistor actually opens up a new pathway through which the charge can pass. Not all the charge goes through all the resistors. By opening a new branch in the circuit, the fraction of charge which passes through any one resistor is reduced. So less charge actually encounters a single resistor. Now it makes sense to say that more resistors (i.e., more branches) leads to less overall resistance.

d. TRUE - If increasing the number of resistors has the effect of decreasing the overall resistance of the circuit, then it must have the effect of increasing the overall current through the circuit. For a constant battery voltage, current and resistance are inversely proportional.

e. FALSE - The overall resistance of a parallel circuit is expressed by the equation:

1/R_Tot = 1/R₁ + 1/R₂ + 1/R₃

So for this circuit, substituting 3 Ohms for each of the resistors leads to a total resistance of 1 Ohm. The total current through the circuit is given by the equation I = (V_Tot) / (R_Tot) = (12 Volts) / (1 Ohm) = 12 Amps.

f. TRUE - In any circuit - whether series or parallel, the voltage gained by the charge in passing through the battery - in this case, 12 Volts - is equal to the overall voltage drop of the charge as it passes through the external circuit. In the case of a parallel circuit, the charge makes an either-or choice between resistors. It passes through a single resistor instead of all three resistors. Thus, it must drop 12 Volts during the pass through that resistor. The voltage drop across each branch is 12 Volts.

g. TRUE - The total or equivalent resistance of these two parallel resistors is simply the ratio of the battery voltage to the overall current. That is, R_Tot = (V_Tot) / (I_Tot) = (12 Volts) / (6 Amps) = 2 Ohms. For resistors in parallel, the overall or total resistance is expressed by the equation

1/R_Tot = 1/R₁ + 1/R₂

Substituting 4 Ohms for each of the individual resistances leads to the overall resistance of 2 Ohms. This is definitely a true statement.

Useful Web Links

Two Types of Connections || Parallel Circuits

Part B: Multiple Choice

8. If an electric circuit was analogous to a water park, then the battery would be analogous to the ____.

a. pipes which carry the water through the water circuit
b. pump which supplies energy to move the water from the ground to a high elevation

c. the people which flow from the top of the water ride to the bottom of the water ride

d. the rate at which water is pumped onto the slide

e. the change in potential energy of the riders

f. the top of the water slide

g. the bottom of the water slide

h. the long lines which exist at the park

i. the speed at which riders move as they slide from the top to the bottom of the ride

Answer: B

A water ride at a water park is analogous to an electric circuit. First of all, there is an entity which flows - water flows in a water park and (in conventional terms) + charge flows in an electric circuit. In each case, the fluid flows spontaneously from a high energy location to a low energy location. The flow is through pipes (or slides) in a water park and through wires in an electric circuit. If the pipes or the wires are broken, then there can be no continuous flow of fluid through the circuit. A complete loop is required to establish the circuit.

This flow of fluid - whether of water or charge - is possible when a pressure difference is created between two locations in the circuit. In the water park, the pressure difference is the difference in water pressure created by two locations of different heights. Water flows spontaneously from locations of high pressure (high altitude) to locations of low pressure (low altitude). In an electric circuit, the electric potential difference between the two terminals of a battery or energy source provides the electric pressure which presses on charge to move them from a location of high pressure (high electric potential) to a location of low pressure (low electric potential).

Energy is required to move the fluid uphill. In a water park, a water pump is used to do work upon the water in order to raise it from the low height back up to the high height. The water pump does not supply the water; the water which is already in the pipes. Rather, the water pump supplies the energy to pump the water from the location of low energy and low pressure to the location of high energy and high pressure. In an electric circuit, the battery is the charge pump which pumps the charge through the battery from the location of low electric potential energy (the - terminal) to the location of high electric potential energy (the + terminal). The battery does not supply the electric charge; the charge is already in the wires. The battery simply supplies the energy to do work on the charge in pumping it uphill.

Useful Web Links

Requirements of a Circuit

9. If an electric circuit was analogous to a water park, then the positive terminal of the battery would be analogous to the ____.

a. pipes which carry the water through the water circuit
b. pump which supplies energy to move the water from the ground to a high elevation

c. the people which flow from the top of the water ride to the bottom of the water ride

d. the rate at which water is pumped onto the slide

e. the change in potential energy of the riders

f. the top of the water slide

g. the bottom of the water slide

h. the long lines which exist at the park

i. the speed at which riders move as they slide from the top to the bottom of the ride

Answer: F

Useful Web Links

Electric Potential Difference || Requirements of a Circuit

10. If an electric circuit was analogous to a water park, then the electric current would be analogous to the ____.

a. pipes which carry the water through the water circuit
b. pump which supplies energy to move the water from the ground to a high elevation

c. the people which flow from the top of the water ride to the bottom of the water ride

d. the rate at which water is pumped onto the slide

e. the change in potential energy of the riders

f. the top of the water slide

g. the bottom of the water slide

h. the long lines which exist at the park

i. the speed at which riders move as they slide from the top to the bottom of the ride

Answer: D

The flow of water at a water park is analogous to the flow of charge in an electric circuit. The rate at which charge moves past a point on a circuit as measured in Coulombs of charge per second (or some comparable set of units) is known as the current. In our analogy, the fluid which flows is water and the rate at which the fluid passes any given point is the current.

Useful Web Links

Electric Current

11. The potential energy possessed per unit of charge at any given location is referred to as the electric ___.

a. current

b. resistance

c. potential

d. power

Answer: C

This is the definition of electric potential - a concept you should internalize.

Useful Web Links

Electric Potential

12. One ampere is the amount of current that exists when ____ flows by a certain point in a conductor in ____.

a. one watt; one second

b. one joule; one hour

c. one electron; one second

d. one electron; one hour

e. one volt; one second

f. one volt; one hour

g. one coulomb; one second

h. one coulomb; one hour

Answer: G

An ampere is a unit of electric current. And electric current is defined as the rate at which charge moves past a point on a circuit as measured in standard units of Coulombs of charge per second.

Useful Web Links

Electric Current

13. If 6 coulombs of charge flow past point 'A' in a circuit in 4 seconds, then ____ coulombs of charge will flow past point 'A' in 8 seconds.

a. 0.67

b. 1.5

c. 2

d. 3

e. 4

f. 6

g. 8

h. 12

i. 24

Answer: H

The current (I) is the quantity of charge flowing past a point (Q) in a given amount of time (t). That is, I = Q/t. So in this case, the current at point A is (6 C) / (4 s) or 1.5 amperes. Thus the Q/t ratio is 1.5 regardless of the time. So solve the equation

1.5 C/s = Q / (8 s)

for Q to obtain the answer.

Useful Web Links

Electric Current

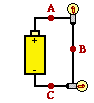
14. In which of the following situations will the light bulb light? List all that apply.

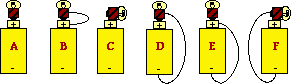
Answer: DF

For a circuit to be established, there must be a closed conducting loop from the positive terminal to the negative terminal. This would mean that circuits D, E and F would all be circuits. But in order for the light bulb to light, it must be included as part of the electric circuit. So in E, the bulb does not light since the loop does not extend up into and through the light bulb; charge would simple flow out of the + terminal battery and directly back into - terminal the battery.

Useful Web Links

Requirements of a Circuit

For Questions #15-#17:

A simple circuit containing a battery and a light bulb is shown in the diagram at the right. Use this diagram to answer the next several questions.

15. The current through the battery is ___.

a. greater than that through the light bulb

b. less than that through the light bulb

c. the same as that through the light bulb

d. greater than that through each wire

e. less than that through each wire

Answer: C

Charge is a conserved quantity; it is never gained nor lost. In an electric circuit, the charge present in the wires and conducting elements is what moves through the circuit. This charge is enclosed in the wires and unable to escape (assuming there is no fault in the circuit). As the charge flows, it does not accumulate in a given location. And charge is not used up as though it were a consumable quantity. Nor is charge transformed into another type of entity. Given all this reasoning, one would conclude that the current at one location in an electric circuit is the same as the current at any other location in an electric circuit.

Useful Web Links

Requirements of a Circuit || Electric Current

16. Charge flowing through this circuit is most energized at ____. Choose the one best answer.

a. the + terminal of the battery
b. the - terminal of the battery

c. just prior to entering the light bulb

d. just after exiting the light bulb

e. ... nonsense! The energy of the charge is the same everywhere throughout the circuit.

Answer: A

The + terminal of the battery is the high energy terminal of the battery.

Useful Web Links

Electric Potential || Electric Potential Difference

17. The role or purpose of the battery in this circuit is to ____. Choose three.

a. supply electric charge so that a current can exist
b. supply energy to the charge

c. move the charge from the - to the + terminal of the battery

d. transform energy from electrical energy into light energy

e. establish an electric potential difference between the + and - terminals

f. replenish the charge which is lost in the light bulb

g. offer resistance to the flow of charge so that the light bulb can get hot

Answer: BCE

To establish an electric circuit, charge must be moved from low energy to high energy. Once at high energy, the charge spontaneously flows through the conducting wires and other conducting elements of the circuit back down to the low energy terminal. A battery's role is to supply the energy which is required to move the charge from the - terminal to the + terminal of the battery. By placing a large quantity of like charge at one location, an electric pressure or potential difference is established, forcing the like charges to move away from this location to the location of opposite charge (the - terminal).

Useful Web Links

Electric Potential Difference || Common Misconceptions Regarding Electric Circuits

18. A 12-Volt battery would supply ___. List all that apply.

a. 3 Coulombs of charge with 4 Joules of energy
b. 4 Coulombs of charge with 3 Joules of energy

c. 12 Coulombs of charge with 1 Joule of energy

d. 1 Coulomb of charge with 12 Joules of energy

e. 0.5 Coulombs of charge with 24 Joules of energy

f. 24 Coulombs of charge with 2 Joules of energy

Answer: D

Electric potential (or voltage) is defined as the electric potential energy per charge. It is the Joules of energy per coulomb of charge possessed by some quantity of charge at some location in an electric circuit. A 12 Volt battery moves some quantity of charge from the - terminal to the + terminal, giving the charge energy. Each coulomb of charge would acquire 12 Joules of energy. The energy/charge ratio would be 12 J/C.

Useful Web Links

Electric Potential Difference

19. The charges that flow through the wires in your home ____.

a. are stored in the outlets at your home
b. are created when an appliance is turned on

c. originate at the power (energy) company

d. originate in the wires between your home and the power company

e. already exist in the wires at your home

Answer: E

This question targets a common misconception about electric circuits. The misconception presumes that the role of the electric outlet, the battery, or the power company is to provide the charge required to move through the home. But the power company is only the source of the energy required to set the charge in motion by the establishment of an electric potential difference. The charge itself is present in the wires and conducting elements of your home in the form of mobile electrons.

Useful Web Links

Common Misconceptions Regarding Electric Circuits

20. Approximately how long would it take an electron to travel from the battery of a car to a head light and back (complete loop)?

a. seconds

b. hours

c. years

d. one-millionth of a second

e. one-tenth of a second

Answer: B

Electric charge drifting through an electric circuit moves at a rather slow pace. Quite surprising to many, the distance traversed per unit of time is on the order of 1 meter per hour.

Useful Web Links

Electric Current || Common Misconceptions Regarding Electric Circuits

21. The electric circuit shown at the right consists of a battery and three identical light bulbs. Which of the following statements are true concerning this circuit? List all that apply.

a. The current through point X will be greater than that through point Z.
b. The current through point Z will be greater than that through point Y.

c. The current will be the same through points X, Y and Z.

d. The current through point X will be greater than that through point Y.

e. The current through point Y will be greater than that through point X.

Answer: C

As discussed in Question #15 above, the current in an electric circuit is everywhere the same. So the current at these three locations is the same.

Useful Web Links

Electric Current || Common Misconceptions Regarding Electric Circuits

22. The electric circuit shown at the right consists of a battery and three identical light bulbs. Which of the following statements are true concerning this circuit? List all that apply.

a. The electric potential difference between X and Y is more than that between Y and Z.
b. The electric potential difference between X and Z is more than that between Y and W.

c. The electric potential difference between X and Y is the same as that between Y and Z.

d. The electric potential difference between X and Z is the same as that between Y and W.

e. The electric potential difference between Y and W is more than that between X and Y.

Answer: DE

The electric potential difference across a light bulb (or any resistor) in an electric circuit is simply the product of the current at that bulb multiplied by the resistance of the bulb. Each bulb has the same resistance (since they're identical) and the same current (since the current is everywhere the same). So the electric potential difference across each bulb is the same. And the potential drop across any two consecutive bulbs is the same. And the potential drop across two bulbs would be greater than that across one bulb.

Useful Web Links

Electric Potential Difference || Common Misconceptions Regarding Electric Circuits

23. The electric circuit shown at the right consists of a battery and three identical light bulbs. Which of the following statements are true concerning this circuit? List all that apply.

a. Conventional current is directed through the external circuit from point X to Y to Z to W.
b. Conventional current is directed through the external circuit from point W to Z to Y to X.

c. Conventional current is directed through the internal circuit from point W to point X.

d. Conventional current is directed through the internal circuit from point X to point W.

e. The point where charge possesses the least amount of electric potential energy is point W.

Answer: ACE

The battery is referred to as the internal circuit. Charge moves in the internal circuit from the - terminal to the + terminal (in the direction from W towards Z). The wires and light bulbs comprise the external circuit; charge moves through the external circuit from the + terminal to the - terminal (in the direction of X to Y to Z to W).

Useful Web Links

Electric Current

24. Voltage ____ an electrical circuit.

a. goes through

b. is expressed across

c. is constant throughout

d. is the rate at which charges move through

Answer: B

Voltage or electric potential is not a thing which moves. Thus, choices A and D are not the answers since they imply movement of voltage. And the voltage or electric potential of a charge is not something which is constant throughout the circuit as choice C suggests.

Voltage or electric potential is a measure of how energized a quantity of charge is at a given location relative to the - terminal. It is often expressed as a difference across two points. Perhaps you have noted this language of "the potential across ..." in several of the answers in this Review.

Useful Web Links

Electric Potential || Electric Potential Difference

25. Two or more of the following words and phrases mean the same thing. Identify them by listing their letters.

a. Voltage

b. Wattage

c. Electric Potential Difference

d. Rate at which charge flows

e. Electric Pressure

f. Energy

Answer: ACE

The voltage or electric potential difference are synonymous terms. Voltage is not synonymous with energy. While voltage (or electric potential difference) is a measure of how energized a quantity of charge is at a given location, voltage is expressed as the energy per charge (and not simply as energy). Drawing from the analogy between a water park and an electric circuit, voltage is a measure of the quantity of electric pressure placed upon a charge in inducing it to move from one location to another location.

Wattage is synonymous with power. Current is synonymous with the rate at which charge flows.

Useful Web Links

Electric Potential || Electric Potential Difference

26. A high voltage battery can ____.

a. do a lot of work on each charge it encounters
b. do a lot of work over the course of its lifetime

c. push a lot of charge through a circuit

d. last a long time

Answer: A

Voltage refers to the energy/charge. A battery rated with a high voltage can do a lot of work per every Coulomb of charge which it encounters. It may or may not be able to do a lot of work over its lifetime, depending upon the size of the battery.

Useful Web Links

Electric Potential Difference

27. Which one of the following occurs when a rechargeable battery is recharged?

a. The battery, which has run out of watts, has its wattage restored.
b. The battery, which has run out of amps, has current placed back into it.

c. The battery, which has run out of charge, has charge returned to it.

d. The battery, which has run out of chemical reactants, has its chemicals reformed.

Answer: D

Batteries perform their energy-supplying tasks by using the energy from an exothermic oxidation-reduction reaction to do work upon charge within the electric circuit. When a battery no longer works, its reactants are consumed to the point that the electric potential which the reactants are capable of producing is small compared to the overall resistance of the circuit. At such an instant in time, the ability to induce a current is limited to the point that the external circuit elements are no longer functional.

Not all batteries are rechargeable. Those that are rechargeable can have the products transformed back into reactants. The recharger utilizes electrical energy from an outlet to reverse the previously exothermic reaction, turning its products back into reactants.

Useful Web Links

Common Misconceptions Regarding Electric Circuits

28. Birds can safely stand on high voltage electric power lines. This is because ____.

a. they are at low potential with respect to the ground.
b. they offer no resistance to current.

c. they always choose power lines that are not in use.

d. the potential difference between their feet is low.

e. they are perfect insulators.

f. they are perfect conductors.

Answer: D

In order for charge to flow between two locations, there must be an electric potential difference established between those two locations. If a bird places its left foot on an electric power line and his right foot a few centimeters away on the same electric power line, then there is little to no difference in potential between his two feet. Without an electric potential difference, charge will not flow through the bird and the bird is safe.

Useful Web Links

Electric Potential Difference || Requirements of a Circuit

29. When the light bulb in your lamp no longer works, it is because the bulb has _____.

a. run out of energy and can no longer pump charge
b. run out of voltage and must be recharged

c. run out of electrons and so there is no more current

d. burned all of its watts and can no longer shine

e. tripped a circuit breaker and must be fixed at the fuse box

f. a broken filament which has resulted in an open circuit

g. ... nonsense! The bulb is fine; your family just needs to fully pay their power bill.

Answer: F

The most common cause for the inability of a light bulbs to light is a broken filament. A coiled wire of tungsten stretches between two vertical supports. If disturbed while hot or if overworn, the tungsten metal can break and leave a gap between the two vertical supports. This gap represents a break in the circuit; a closed conducting loop is no longer established and charge will not flow.

Useful Web Links

Requirements of a Circuit

30. A battery is needed in the circuit of your flashlight so that ____.

a. charge is provided to the wires
b. the energy of the light is balanced by the battery

c. an exothermic, light-creating reaction is possible

d. an electric potential difference is maintained across the circuit

e. electrons are supplied in order to light the bulb

Answer: D

One of the roles of a battery is to simply establish a difference in electric potential between its two terminals. Charge at the high potential will flow through the external circuit to the low potential location.

Useful Web Links

Requirements of a Circuit || Common Misconceptions Regarding Electric Circuits

31. When you turn on the room lights, they light immediately. This is best explained by the fact that ____.

a. electrons move very fast from the switch to the light bulb filament
b. electrons present everywhere in the circuit move instantly

Answer: B

Electrons move very slowly from one location to another location. But once a circuit is closed, they start moving immediately. While electrons move about a meter or in an hour, the actual signal which tells them to start moving can travel at the speed of light. So once the switch is turned on, a signal is circulated throughout the circuit to start the electrons marching. The electrons present in the filament of the circuit.

Useful Web Links

Common Misconceptions Regarding Electric Circuits

32. The drift velocity of mobile charge carriers in electric circuits is ____.

a. very fast; less than but very close to the speed of light
b. fast; faster than the fastest car but nowhere near the speed of light

c. slow; slower than Michael Jackson runs the 220-meters

d. very slow; slower than a snail

Answer: D

Drift velocity is the distance which a charge moves per unit of time. This value is very small since electrons move very, very slowly. Moving at about 1 meter per hour, they are literally slower than a snail.

Useful Web Links

Electric Current || Common Misconceptions Regarding Electric Circuits

33. Suppose that the current in a typical circuit (DC) is large. This is an indication that ____.

a. the mobile charge carriers are moving very fast
b. a large number of mobile charge carriers are moving forward per second

c. both a and b are true

Answer: B

Current (the rate at which charge moves past a point on the circuit) and drift velocity (the distance a charge moves in a second) should not be confused (and often are). If a current is large, one can be sure of only one thing: a lot of charges are moving forward past a point on the circuit every second.

Useful Web Links

Electric Current

34. Which of the following statements represent correct unit equivalencies? List all that apply.

a. 1 Ampere = 1 Coulomb / second

b. 1 Joule = 1 Volt / Coulomb

c. 1 Watt = 1 Joule • second

d. 1 Watt = 1 Volt • Coulomb / second

e. 1 Joule / Ohm = 1 Ampere • Coulomb

f. 1 Joule • Ohm = 1 Volt² • second

Answer: ADEF

This question requires a knowledge of both units for electrical quantities and the equations which relate those quantities.

In choice a, an Ampere is a unit of current (I) and a Coulomb/second is a unit of charge per unit of time (Q/t). This is consistent with the equation I = Q/t.

In choice b, a Joule is a unit of energy (E) and a Volt / Coulomb is a unit of voltage per unit of charge (V/Q). Since voltage is the energy per charge, we would expect that energy would be equivalent to voltage • charge. Thus, it is incorrect to equate units of energy to units of voltage per charge.

In choice c, a Watt is a unit of power (P) and a Joule•second is a unit of energy (E) multiplied by a unit of time (t). But power is energy / time and not energy • time so this is not a correct unit equivalency.

In choice d, a Watt is a unit of power (P). On the right side, a Volt is a unit of voltage (V) and a Coulomb / second is a unit of current (I). So since P = I • V, this is a correct unit equivalency.

In choice e, a Joule / Ohm is a unit of energy per unit of resistance (E / R). An Ampere • Coulomb is a unit of current multiplied by a unit of charge (I • Q). Thus, the equation is suggesting that E / R = I • Q. This can be rearranged algebraically to say that E / Q = I • R. Since voltage is the energy per charge (E / Q), the equation can be rewritten as V = I • R. This is thus a correct unit equivalency.

In choice f, a Joule• Ohm is a unit of energy multiplied by a unit of resistance (E • R). A Volt² / second is a unit of voltage² multiplied by a unit of time (V² • t). So this equation is suggesting that E • R = V² • t. This can be rearranged algebraically to say that E / t = V² / R. The right side of the equation is equivalent to power, so the equation can be rewritten as P = V² / R. Since this is a correct way of writing the power equation, the given unit equivalency is correct.

Useful Web Links

Electric Current || Power Revisited

35. Which of the following diagrams represents resistors connected in in series? List all that apply.

Answer: B

A and C represent parallel connections as shown by the branching which occurs before and after the resistors. There is no branching in choice B so it is a series connection of resistors.

Useful Web Links

Two Types of Connections

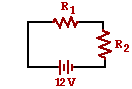
Questions #36-#39:

The diagram at the right shows two identical resistors - R₁ and R₂ - placed in a circuit with a 12-Volt battery. Use this diagram to answer the next several questions.

36. These two resistors are connected in ____.

a. series

b. parallel

c. neither

Answer: A

Useful Web Links

Two Types of Connections

37. The electric potential difference (voltage drop) across each resistor is ___ Volts.

a. 6

b. 12

c. 24

d. ... nonsense!. The electric potential difference is dependent upon the actual resistance of the resistors

Answer: A

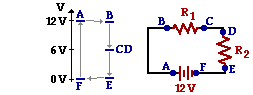Charge receives an increase in electric potential of 12 volts when moving through the internal circuit (the battery). So as a charge leaves the battery and traverses the external circuit, there must be a total drop in electric potential of 12 Volts. This drop in voltage occurs in a two-step fashion as the charge passes through each of the resistors. The charge will lose 6 volts in the first resistor and 6 volts in the second resistor, bringing it back to zero volts by the time it returns to the - terminal of the battery. The potential diagram at the right is a visual means of representing this important concept.

Useful Web Links

Series Circuits

38. If a third resistor (R₃), identical to the other two, is added in series with the first two, then the overall resistance will ____ and the overall current will ____.

a. increase, increase

b. decrease, decrease

c. increase, decrease

d. decrease, increase

e. increase, remain the same

f. decrease, remain the same

g. remain the same, increase

h. remain the same, decrease

i. remain the same, remain the same

Answer: C

Increasing the number of resistors in a series circuit will increase the overall resistance of that circuit and cause the current to decrease. (The opposite is true of a parallel circuit.)

Useful Web Links

Series Circuits

39. If a third resistor (R₃), identical to the other two, is added in series with the first two, then the electric potential difference (voltage drop) across each of the three individual resistors will ____.

a. increase

b. decrease

c. remain the same

Answer: B

Using the same reasoning as in Question #37, we can say that the charge will acquire 12 Volts in moving through the battery. It will have to lose this 12 volts in three steps as it passes through the external circuit. Since there are now three voltage drops in the external circuit instead of the original two, each drop must be smaller than before. So there will be a 4-Volt drop through each resistor (instead of the original 6-Volt drop).

Useful Web Links

Series Circuits

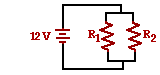Questions #40-#43:

The diagram at the right shows two identical resistors - R₁ and R₂ - placed in a circuit with a 12-Volt battery. Use this diagram to answer the next several questions.

40. These two resistors are connected in ____.

a. series

b. parallel

c. neither

Answer: B

One can start at the positive terminal of the battery and begin tracing their finger along the wire. If there is ever a point where the wire comes to a junction and branches in two or more directions, then the circuit has a parallel connection. Otherwise, it is a series circuit. In this diagram, there is some branching. Once the charge reaches the branching point, it will either pass through the resistor in the left branch (R₁) or through the resistor in the right branch (R₂). Thus, it is a parallel circuit.

Useful Web Links

Parallel Circuits

41. The electric potential difference (voltage drop) across each resistor is ___ Volts.

a. 6

b. 12

c. 24

d. ... nonsense!. The electric potential difference is dependent upon the actual resistance of the resistors

Answer: B

Charge receives an increase in electric potential of 12 volts when moving through the internal circuit (the battery). So as a charge leaves the battery and traverses the external circuit, there must be a total drop in electric potential of 12 Volts. This drop in voltage occurs in a single step since the charge will only pass through a single resistor on its path back to the battery. So since the charge chooses either the left or the right branch (and not both), either branch must provide the 12-Volt drop in voltage. In parallel circuits, the the electric potential difference across the battery is equal to the electric potential difference across either branch. The potential diagram at the right is a visual means of representing this important concept.

Useful Web Links

Parallel Circuits

42. If a third resistor (R₃), identical to the other two, is added in parallel with the first two, then the overall resistance will ____ and the overall current will ____.

a. increase, increase

b. decrease, decrease

c. increase, decrease

d. decrease, increase

e. increase, remain the same

f. decrease, remain the same

g. remain the same, increase

h. remain the same, decrease

i. remain the same, remain the same

Answer: D

Adding an identical resistor in a separate branch will provide more pathways by which charge can traverse through the loop of the circuit. This would be the equivalent of adding another booth at a toll station on a tollway in parallel with an existing booth. Opening up another lane for traffic will reduce the overall resistance and cause an increase in the car flow rate. The same occurs with charge in parallel circuits. More branches means less resistance and an increased current.

Useful Web Links

Parallel Circuits

43. If a third resistor (R₃), identical to the other two, is added in parallel with the first two, then the electric potential difference (voltage drop) across each of the three individual resistors will ____.

a. increase

b. decrease

c. remain the same

Answer: C

The electric potential difference across any branch is equal to the voltage of the battery. Adding a new branch can alter the overall resistance and the overall current, but it does not alter the electric potential difference across the battery nor across the branches.

Useful Web Links

Parallel Circuits

44. The resistance of a charge-carrying conducting wire will increase as the ____. Choose all that apply.

a. length of the wire is increased
b. cross-sectional area of the wire is increased

c. temperature of the wire is increased

d. voltage impressed across the ends of the wire is increased

e. wire is placed closer and closer to the + terminal of the circuit

Answer: AC

Resistance of a wire increases with increasing length and (to a smaller extent) with increasing temperature. Increasing wire length increases the number of atom-charge collisions and thus the amount of resistance. Increasing the temperature increases the resistivity of the material and thus increases the overall resistance.

Useful Web Links

Resistance

45. When plugged into a 120-Volt outlet, a light bulb consumes 300 joules of energy over a 5 second time period. The power of the light bulb is ____ Watts.

a. 0.0167

b. 0.50

c. 2.0

d. 2.50

e. 60

f. 600

g. 1500

h. 7200

Answer: E

Power is simply the rate at which energy is supplied to a circuit or transformed by a circuit. In this case, the power is the energy consumed per time.

P = (300 J) / (5 seconds) = 60 Watts

Useful Web Links

Power: Putting Charges to Work

46. A certain electrical circuit contains a battery, wires and a light bulb. If potential energy is gained by charges at the battery location, then charges lose potential energy ____.

a. in the wires only
b. in the bulb only

c. equally in the wires and the bulb

d. mostly in the wires but a little in the bulb

e. mostly in the bulb but a little in the wires

f. nowhere

Answer: E

Charge will lose energy as they pass through locations of resistance. When in series, locations of greatest resistance will transform electrical energy into other forms at a greater rate. So energy will be lost in the light bulb and in the wires to a much lesser extent.

Useful Web Links

Journey of a Typical Electron

47. A high resistance light bulb and a low resistance light bulb are connected in series to a 6-Volt pack of batteries. Which of the two light bulbs will shine the brightest?

a. They will have the same brightness.
b. The low-R bulb will shine more brightly.

c. The high-R bulb will shine more brightly.

d. There is no way to make such a prediction since bulb brightness is independent of bulb resistance.

Answer: C

Since the two light bulbs are in series, the same current (i) is experienced by each. The power will be given by the i²•R product. Since i is the same for each light bulb, the bulb with the greatest resistance will have the greatest power. So the high-R light bulb will transform electrical energy into light energy at the greatest rate and thus shine most brightly.

Useful Web Links

Series Circuits

48. A high resistance light bulb and a low resistance light bulb are connected in parallel and powered by a 6-Volt pack of batteries. Which of the two light bulbs will shine the brightest?

a. They will have the same brightness.
b. The low-R bulb will shine more brightly.

c. The high-R bulb will shine more brightly.

d. There is no way to make such a prediction since bulb brightness is indepenent of bulb resistance.

Answer: B

Since the two light bulbs are in parallel, the same voltage drop (V) is experienced by each. The power will be given by the i²•R product. Since V is the same for each light bulb, the bulb with the greatest resistance will have the lest current. Current is of the greatest importance in determining the power of the light bulb since it is squared in the equation. So the low-R light bulb will have the greatest current and thus transform electrical energy into light energy at the greatest rate; it will shine most brightly.

Useful Web Links

Parallel Circuits

49. Three identical light bulbs are connected to a battery as shown at the right. Which adjustments could be made to the circuit that would increase the current being measured at X? Include all that apply.

a. increase the resistance of one of the bulbs
b. increase the resistance of two of the bulbs

c. decrease the resistance of two of the bulbs

d. increase the voltage of the battery

e. decrease the voltage of the battery

f. remove one of the bulbs

Answer: CDF

The current in a series circuit (both total current and current through individual resistors) is directly dependent upon the battery voltage and inversely dependent upon the total circuit resistance. This current can be increased by increasing the battery voltage. It can also be increased by decreasing the total resistance. Removing a bulb would decrease the total resistance and decreasing the resistance of any individual bulb would decrease the total resistance.

Useful Web Links

Series Circuits

50. Three identical light bulbs (labeled X, Y and Z) are connected to a battery as shown at the right. Which adjustments could be made to the circuit below that would increase the current at point P? List all that apply.

a. increase the resistance of one of the bulbs
b. increase the resistance of two of the bulbs

c. decrease the resistance of two of the bulbs

d. increase the voltage of the battery

e. decrease the voltage of the battery

f. remove one of the bulbs

Answer: CD

Point P represents the location where the total current of this parallel circuit can be measured. The total current would vary directly with the total voltage and inversely with the total resistance. Increasing the battery voltage would increase the current at location P. Decreasing the total resistance would increase the current at location P. The total resistance can be decreased by adding another resistor in a separate branch or by decreasing the resistance of any of the branches.

Useful Web Links

Parallel Circuits

51. Three identical light bulbs (labeled X, Y and Z) are connected to a battery as shown at the right. Which adjustments could be made to the circuit below that would decrease the current in bulb Z? List all that apply.

a. increase the resistance of bulb X
b. decrease the resistance of bulb X

c. increase the resistance of bulb Z

d. decrease the resistance of bulb Z

e. increase the voltage of the battery

f. decrease the voltage of the battery

g. remove bulb Y

Answer: CF

The current in bulb Z is dependent upon the voltage drop across bulb Z and the resistance of bulb Z. In equation form,

I_Z = V_Z / R_Z

An increase in battery voltage would increase the voltage drop across bulb Z (V_Z) and thus provide a greater current through the bulb. A decrease in the resistance of bulb Z would also increase the current through the bulb. However, making a change in bulb X or Y would have no effect on the V_Z / R_Z ratio.

Useful Web Links

Parallel Circuits

Part C: Diagramming and Analysis

52. Consider the diagram at the right to answer the following questions.

a. If 4 Coulombs of charge flow past point A in 2 seconds, then ___ Coulombs of charge flow past point B in 2 seconds.

a. less than 4

b. 4

c. more than 4

d. impossible to make such a prediction without knowledge of the resistances.

b. If 4 Coulombs of charge flow past point A in 2 seconds, then ___ Coulombs of charge flow past point B in 1 second.

a. less than 4

b. 4

c. more than 4

d. impossible to make such a prediction without knowledge of the resistances.

c. If 4 Coulombs of charge flow past point A in 2 seconds, then ___ Coulombs of charge flow past point B in 4 seconds.

a. less than 4

b. 4

c. more than 4

d. impossible to make such a prediction without knowledge of the resistances.

d. If 4 Coulombs of charge flow past point A in 2 seconds, then ___ Coulombs of charge flow past point B in 4 seconds.

e. If 4 Coulombs of charge flow past point A in 2 seconds, then ___ Coulombs of charge flow past point C in 4 seconds.

f. Suppose that the resistance of the light bulb located between points A and B is increased. This would cause the current through the other light bulb to ____ (increase, decrease, remain the same).

Answer: See answers below.

This question tests your understanding of current as the rate at which charge (expressed here in Coulombs) flows past a point on a circuit. Current is found as the charge/time ratio. For a series circuit such as this one, the current is everywhere the same.

a. B; the current is 2.0 amperes at point A. To be the same 2.0 Amperes at point B, 4 Coulombs must pass the point in 2 seconds.

b. A; the current is 2.0 amperes at point A. To be the same 2.0 Amperes at point B, 2 Coulombs must pass the point in 1 second. In a lesser amount of time, less charge will flow past the point.

c. C; the current is 2.0 amperes at point A. To be the same 2.0 Amperes at point B, 8 Coulombs must pass the point in 4 seconds. In a greater amount of time, more charge will flow past the point.

d. 8; the current is 2.0 amperes at point A. To be the same 2.0 Amperes at point B, 8 Coulombs must pass point B in 4 seconds.

e. 8; the current is 2.0 amperes at point A. To be the same 2.0 Amperes at point B, 8 Coulombs must pass point C in 4 seconds.

f. decrease; increasing the resistance of a light bulb for a series of consecutive light bulbs will increase the overall resistance of the circuit and cause the current throughout the entire circuit to decrease.

Useful Web Links

Electric Current || Series Circuits

53. The diagram at the right shows three identical light bulbs wired in series. Several points along the circuit are labeled with letters. Compare the electric potential and the electric potential energy of the various points. For each comparison, use a greater than (>), less than (<), or approximately equal to (=) symbol.

Electric Potential
Comparison
Potential Energy
Comparison

V_A = V_B PE_A = PE _B

V_B > V_C PE _B > PE _C

V_C = V_D PE _C = PE _D

V_D > V_E PE _D > PE _E

V_E = V_F PE _E = PE _F

V_F > V_G PE _F > PE _G

V_G = V_H PE _G = PE _H

V_H < V_A PE _H < PE _A

Answer: See table above.

Point A corresponds to the positive terminal of the battery. At point A, charge possesses the greatest amount of electric potential energy. It has a high voltage at this location. In the process of moving through the circuit to the - terminal, the charge will have its electric potential energy converted to light energy (and heat energy) in the light bulbs. It will lose a portion of its electrical energy in each light bulb. The light bulb simply serves as an energy conversion device which transforms electric potential energy into non-electrical forms. As such, the potential energy (and thus the voltage) will drop in value every time the passes by a light bulb and reaches a point further from the high energy + terminal. Finally, at point H, all the electrical energy and voltage has been lost and the charge is in need of some energy input from the battery.

Useful Web Links

Electric Potential Difference || Series Circuits

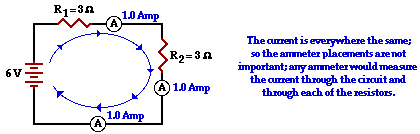
54. Use proper schematic symbols to construct a diagram of a circuit powered by a 6-Volt battery that consists of two 3-ohm resistors connected in series. Place ammeters in series at a location such that the current through each resistor can be measured and in a location such that the overall current in the circuit can be measured. On the schematic diagram, use an unbroken arrow to indicate the direction of conventional current. Finally, indicate the ammeter readings on the diagram.

Answer: See diagram below.

Since the two resistors are in series, the total or equivalent resistance is simply the sum of the individual resistances. The total resistance is 6 ohms. The total current in the circuit can be found by the ratio of battery voltage to total resistance:

I_TOT = ΔV_TOT / R_TOT = (6 V) / (6 ohms) = 1 Amp

Since it is a series circuit, the current through the battery is the same as the current through each of the resistors.

Useful Web Links

Circuit Symbols and Circuit Diagrams || Series Circuits

55. Use proper schematic symbols to construct a diagram of a circuit powered by a 6-Volt battery that consists of two 3-ohm resistors connected in parallel. Place an ammeter in series with each of the individual resistors in a manner that the current through each resistor can be measured. Place a third ammeter in a location such that the overall current in the circuit can be measured. On the schematic diagram, use an unbroken arrow to indicate the direction of conventional current. Finally, indicate the ammeter readings on the diagram.

Answer: See diagram below.

The voltage across each branch is equivalent to the voltage of the battery. The current through a branch is simply the V/R ratio where V = 6 Volts and R = 3 ohms. This calculation leads to the conclusion that 2 Amps is the current in each branch. The current outside the branches is simply the sum of the current in the branches. So there will be a current of 4 Amps outside the branches.

As an alternative to this solution, the equivalent resistance could first be determined using the 1/R_Tot equation for parallel circuits. The equivalent resistance is 1.5 Ohms. Once determined, the total circuit current can be calculated using I_Tot = ΔV_Tot / R_Tot. The total circuit current would be 4 Amps. Then divide the 4 Amps into two equal branch currents to determine the current at each of the individual resistors.

Useful Web Links

Circuit Symbols and Circuit Diagrams || Parallel Circuits

56. Consider the diagram at the right of a series circuit. Each light bulb in the circuit has an identical resistance. Use the labeled points on the diagram to answer the following questions. Each question may have one, less than one, or more than one answer.

a. The electric potential at point A is the same as the electric potential at point(s) B. Include all that apply, if any apply.

b. The electric potential at point C is the same as the electric potential at point(s) D and E. Include all that apply, if any apply.

c. The electric potential at point F is the same as the electric potential at point(s) G. Include all that apply, if any apply.

d. The electric potential at point I is the same as the electric potential at point(s) H. Include all that apply, if any apply.

e. The electric potential difference between points A and B is the same as the electric potential difference between points C and D (and C and E; and D and E; and F and G; and H and I). Include all that apply, if any apply.

f. The electric potential difference between points A and C is the same as the electric potential difference between points E and F (and C and F; and D and F; and E and G; and C and G; and D and G; and G and H; and F and H; and G and I; and F and I). Include all that apply, if any apply.

g. The electric potential difference between points A and F is the same as the electric potential difference between points E and H (and between any other two sets of points that are on the opposite side of two adjacent bulbs ... such as points C and H or points D and H or points E and I, etc.). Include all that apply, if any apply.

h. The electric potential difference between points D and H is the same as the electric potential difference between points A and F (and between any other two sets of points that are on the opposite side of two adjacent bulbs ... such as points C and H or points D and I or points E and I, etc.). Include all that apply, if any apply.

i. The current at point A is the same as the current at point(s) C (and at every other point on the circuit). Include all that apply, if any apply.

j. The current at point E is the same as the current at point(s) F (and at every other point on the circuit). Include all that apply, if any apply.

k. The current at point G is the same as the current at point(s) G (and at every other point on the circuit). Include all that apply, if any apply.

Answer: See answers above.

In this circuit, the light bulbs have the same resistance; thus, each light bulb causes the same drop in potential (electric potential difference). So the electric potential difference will be the same between any two points which are distanced by a light bulb, or by two light bulbs. (e through h)

Finally, in a series circuit the current is the same at every point along the circuit. Since charge is conserved and since there is no location in a circuit where the charge is accumulating, there must be the same charge flow rate at all locations. (i through k)

Useful Web Links

Series Circuits

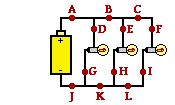57. Consider the diagram at the right of a parallel circuit. Each light bulb in the circuit has an identical resistance. Use the labeled points on the diagram to answer the following questions. Each question may have one, less than one, or more than one answer.

a. The electric potential at point A is the same as the electric potential at point(s) B (and at any other point before the light bulbs ... such as at points C, D, E and F). Include all that apply, if any apply.

b. The electric potential at point D is the same as the electric potential at point(s) B (and at any other point before the light bulbs ... such as at points A, C, E and F). Include all that apply, if any apply.

c. The electric potential at point J is the same as the electric potential at point(s) K (and at any other point after the light bulbs ... such as at points G, H, I, and L. Include all that apply, if any apply.

d. The electric potential difference between points A and J is the same as the electric potential difference between points B and K (and any other combination of two points located on opposite sides of the light bulbs ... such as points A and K, or points A and L, or points F and G, or points D and G, etc., etc.). Include all that apply, if any apply.

e. The electric potential difference between points D and G is the same as the electric potential difference between points A and J (and any other combination of two points located on opposite sides of the light bulbs ... such as points A and K, or points A and L, or points F and G, or points E and H, etc., etc.). Include all that apply, if any apply.

f. The current at point A is the same as the current at point(s) J. Include all that apply, if any apply.

g. The current at point B is the same as the current at point(s) K. Include all that apply, if any apply.

h. The current at point C is the same as the current at point(s) L (and points F and I and E and H and D and G). Include all that apply, if any apply.

i. The current at point D is the same as the current at point(s) G (and at points F and I and C and E and H). Include all that apply, if any apply.

j. If the light bulb located between points D and G were to be replaced by a bulb of greater resistance, then the current at point(s) A, D, G, and J would be decreased. Include all that apply, if any apply.

k. If the light bulb located between points D and G were to be replaced by a bulb of greater resistance, then the electric potential difference between points -- and -- would be increased. Include all that apply, if any apply. (None apply.)

l. If the light bulb located between points D and G were to go out, then the current would decrease at point(s) A, D, G and J. Include all that apply, if any apply.

Answer: See answers above.

In an electric circuit, the electric potential for a moving charge is gained in the battery and lost in a light bulb (or some resistor found in the external circuit). So the electric potential of a charge is the same for any two points which are not separated by a battery or by a light bulb. Even if the circuit is a parallel circuit, any point between the positive terminal of a battery and light bulb will have the same electric potential; and any point located between the - terminal of the battery and a location after passage through the resistor of a branch have the same electric potential. (a through e)

In this circuit, the light bulbs have the same resistance. Thus, when charge reaches the branching location an equal amount of charge will chose the middle branch as does the left branch and the right branch. So with the same resistance, the current in every branch is the same. And the current prior to the branching location and after the branches come together is the same. Finally, locations B and K are at locations where current for two of the three branches will be passing; these points will have the same current. (e through i)

In j, removing the bulb in the first branch will not affect the current in the other branches. Such a modification will only reduce the overall circuit current. Less branches would result in more overall resistance and less overall current. Yet the current through the second branch is still the voltage drop across the second branch (which is the battery voltage) divided by the resistance of the second branch. Since removing the bulb in the first branch does not alter either quantity, the current in the middle branch is not altered.

In k, the electric potential across a branch is simply equal to the voltage of the battery. Removing a light bulb will not alter the voltage of the battery. Thus, there is no effect.

In l, if the light bulb in the first branch burns out, the same effect will occur as occurred in part j.

Useful Web Links

Parallel Circuits

58. Consider the diagram below of a series circuit. For each resistor, use arrows to indicate the two locations where one would have to tap with the leads of a voltmeter in order to measure the voltage drop across the individual resistor. Finally, indicate the ammeter readings and the voltage readings.

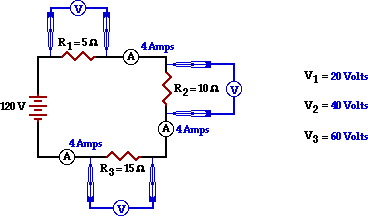
Answer: See diagram above.

The total resistance (or equivalent resistance) can first be determined using the equation for series circuits.

R_Tot = R₁ + R₂ + R₃ = 5 Ohms + 10 Ohms + 15 Ohms = 30 Ohms

Once known, the R_Tot value can be used with the battery voltage (ΔV_Tot) to determine the total current in the circuit.

I_Tot = (ΔV_Tot) / (R_Tot) = (120 V) / (30 Ohms) = 4 Amps

For a series circuit, the current through each resistor is the same as the total circuit current. Thus, I₁ = I₂ = I₃ = 4 Amps.

The voltage drop across a resistor can be determined with a voltmeter by tapping with the leads on the metal wires on the opposite sides of the resistor. By so doing, the voltmeter determines the difference in voltage (i.e., voltage drop or electric potential difference) between the two locations where the leads were tapped. In this circuit, the expected voltage drops (ΔV₁, ΔV₂, and ΔV₃) can be computed by determining the IR product for each resistor. This is shown below.

ΔV₁ = I₁ • R₁ = (4 Amps) • (5 Ohms) = 20 Volts

ΔV₂ = I₂ • R₂ = (4 Amps) • (10 Ohms) = 40 Volts

ΔV₃ = I₃ • R₃ = (4 Amps) • (15 Ohms) = 60 Volts

Useful Web Links

Series Circuits

59. Consider the diagram below of a parallel circuit. For each resistor, use arrows to indicate the two locations where one would have to tap with the leads of a voltmeter in order to measure the voltage drop across the individual resistor. Finally, indicate the ammeter readings and the voltage readings.

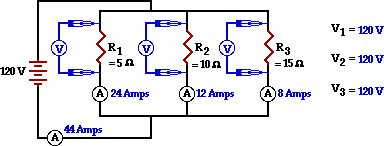
Answer: See diagram above.

The total resistance (or equivalent resistance) can first be determined using the equation for series circuits.

1 / R_Tot = 1 / R₁ + 1 / R₂ + 1 / R₃ = 1 / (5 Ohms + 1 / (10 Ohms) + 1 / (15 Ohms)

R_Tot = 2.727 Ohms

Once known, the R_Tot value can be used with the battery voltage (ΔV_Tot) to determine the total current in the circuit.

I_Tot = (ΔV_Tot) / (R_Tot) = (120 V) / (2.727 Ohms) = 44.0 Amps

The voltage drop across a resistor can be determined with a voltmeter by tapping with the leads on the metal wires on the opposite sides of the resistor. By so doing, the voltmeter determines the difference in voltage (i.e., voltage drop or electric potential difference) between the two locations where the leads were tapped. For a series circuit, the expected voltage drop across each resistor (ΔV₁, ΔV₂, and ΔV₃) is the same as the total voltage drop. Thus, ΔV₁ = ΔV₂ = ΔV₃ = 120 Volts.

In this circuit, the branch currents can be computed by using the ΔV = I•R equation for each resistor. This is shown below.

R₁ = ΔV₁ / R₁ = (120 Volts) / (5 Ohms) = 24 Amps

I₂ = ΔV₂ / R₂ = (120 Volts) / (10 Ohms) = 12 Amps

I₃ = ΔV₃ / R₃ = (120 Volts) / (15 Ohms) = 8 Amps

Useful Web Links

Parallel Circuits

Part D: Qualitative Relationships Between Variables

60. A resistor with a resistance of R is connected to a battery with a voltage of V to produce a current of I. What would be the new current (in terms of I) if ...

a. ... the resistance is doubled and the same voltage is used?
b. ... the voltage is doubled and the same resistance is used?

c. ... the voltage is tripled and the resistance is doubled?

d. ... the voltage is doubled and the resistance is halved?

e. ... the voltage is halved and the resistance is doubled?

f. ... five times the voltage and one-third the resistance is used?

g. ... one-fifth the voltage and one-fourth the resistance is used?

Answer: See answers below.

This question tests your understanding of the current-voltage-resistance relationship. The current is directly proportional to the voltage and inversely proportional to the resistance. Any alteration in the voltage will result in the same alteration of the current. So doubling or tripling the voltage will cause the current to be doubled or tripled. On the other hand, any alteration in the resistance will result in the opposite or inverse alteration of the current. So doubling or tripling the resistance will cause the current to be one-half or one-third the original value.

a. The new current will be 0.5 • I.
b. The new current will be 2 • I.

c. The new current will be 1.5 • I.

d. The new current will be 4 • I.

e. The new current will be 0.25 • I.

f. The new current will be 15 • I.

g. The new current will be 0.8 • I.

Useful Web Links

Ohm's Law

61. A wire of length L and cross-sectional area A is used in a circuit. The overall resistance of the wire is R. What would be the new resistance (in terms of R) if ...

a. ... the length of the wire is doubled?
b. ... the cross-sectional area of the wire is doubled?

c. ... the length of the wire is doubled and the cross-sectional area of the wire is doubled?

d. ... the length of the wire is tripled and the cross-sectional area of the wire is doubled?

e. ... the length of the wire is halved and the cross-sectional area of the wire is tripled?

f. ... the length of the wire is tripled and the cross-sectional area of the wire is halved?

g. ... the length of the wire is tripled and the diameter of the wire is halved?

h. ... the length of the wire is tripled and the diameter of the wire is doubled?

Answer: See answers below.

This question tests your understanding of the variables which effect the resistance of a wire. The resistance of a wire expressed by the equation R = Rho • L / A (where Rho is the resistivity of the material, L is length of wire, and A is cross-sectional area of the wire). The resistance is directly proportional to the resistivity, directly proportional to the wire length, and inversely proportional to the cross-sectional area. Any alteration in the resistivity or the length will result in the same alteration in the resistance of the wire. And any alteration in the cross-sectional area of the wire will result in the opposite or inverse alteration in the resistance of the wire.

a. The new resistance will be 2•R.
b. The new resistance will be 0.5•R.

c. The new resistance will still be R.

d. The new resistance will be 1.5•R.

e. The new resistance will be (1/6)•R.

f. The new resistance will be 6•R.

g. The new resistance will be 12•R. (Halving the diameter will make the area one-fourth the size since area is directly proportional to the square of the diameter.)

h. The new resistance will be (3/4)•R. (Doubling the diameter will make the area four times the size since area is directly proportional to the square of the diameter.)

Useful Web Links

Resistance

62. An electric appliance with a current of I and a resistance of R converts energy to other forms at a rate of P when connected to a 120-Volt outlet. What would be the new power rating (in terms of P) if ...

a. ... the current is doubled (and the same 120-Volt outlet is used)?
b. ... the current is halved (and the same 120-Volt outlet is used)?

c. ... the resistance is doubled (and the same 120-Volt outlet is used)?

d. ... the resistance is halved (and the same 120-Volt outlet is used)?

e. ... the current is tripled (and the same 120-Volt outlet is used)?

f. ... the resistance is tripled (and the same 120-Volt outlet is used)?

g. ... the same appliance is powered by a 12-Volt supply?

h. ... the same appliance is powered by a 240-Volt supply?

Answer: See answers below.

This question tests your understanding of the mathematical relationship between power, current, voltage and resistance. There are three equations of importance:

P = I • ΔV

P = ΔV² / R

P = I² • R

One must be careful in using the last equation since an alteration in current will also alter the resistance whenever the voltage is held constant. Thus, the first two equations are of greater importance since they represent equations with one independent variable and the other variable held constant.

a. 2 • P (doubling the current will double the power)
b. (1/2) • P (halving the current will double the power)

c. (1/2) • P (doubling the resistance will half the power)

d. 2 • P (halving the resistance will double the power)

e. 3 • P (tripling the current will triple the power)

f. (1/3) • P (tripling the resistance will make the power one-third of the original value)

g. (1/100) • P (one-tenth the voltage will make the power one-hundredth of the original value; observe the square on voltage)

h. 4 • P (two times the voltage will make the power four times the original value; observe the square on voltage)

Useful Web Links

Power Revisited

63. An electric appliance with a current of I and a resistance of R is used for t hours during the course of a month. The cost of operating the appliance at 120-Volts is D dollars. What would be the new cost (in terms of D) if ...

a. ... the usage rate was doubled to 2t?
b. ... the usage rate was halved?

c. ... an appliance which drew twice the current (at 120 Volts) were used?

d. ... an appliance with twice the resistance (at 120 Volts) were used?

e. ... an appliance with one-half the resistance (at 120 Volts) were used?

f. ... the usage rate was doubled and an appliance with twice the resistance (at 120 Volts) were used?

g. ... the usage rate was halved and an appliance with twice the current (at 120 Volts) were used?

h. ... the usage rate was quartered and an appliance with twice the current (at 120 Volts) were used?

Answer: See answers below.

Like the previous question, this question tests your understanding of the mathematical relationship between power, current, voltage and resistance. But this question also tests your understanding between power, time, energy and electricity costs. An electric bill is based upon energy consumption. The energy consumed is measured in terms of kiloWatts•hour and is determined by multiplying the power by the time. Thus, an increase in either time or power will lead to an increase in the electricity costs by the same factor. So the key to the question is to use information about power and about usage rate to determine the energy consumed and thus the electricity costs. There are two equations of importance in predicting how alterations in current and resistance effect the power:

P = I • ΔV

P = ΔV² / R

The first equation shows that the power would increase by the same factor by which the current is increased. The second equation shows that the power would decrease by the same factor that the resistance is increased.

a. The new cost would be 2•D.
b. The new cost would be (1/2)•D.

c. The new cost would be 2•D.

d. The new cost would be (1/2)•D.

e. The new cost would be 2•D.

f. The new cost would still be D.

g. The new cost would still be D.

h. The new cost would be (1/2)•D.

Useful Web Links

Power: Putting Charges to Work || Power Revisited

64. Consider the diagram at the right of a parallel circuit. Each light bulb has an identical resistance of R and the battery voltage is V. Use the labeled points on the diagram to answer the following questions.

a. If the current at location A is I amperes, then the current at location B is ____ amperes. (Answer in terms of I.)

b. If the current at location A is I amperes, then the current at location D is ____ amperes. (Answer in terms of I.)

c. If the current at location A is I amperes, then the current at location L is ____ amperes. (Answer in terms of I.)

d. If the voltage of the battery is doubled, then the current at location A would be ____ (two times, four times, one-half, one-fourth, etc.) the original value.

e. If the voltage of the battery is doubled, then the current at location B would be ____ (two times, four times, one-half, one-fourth, etc.) the original value.

f. If the voltage of the battery is doubled, then the current at location D would be ____ (two times, four times, one-half, one-fourth, etc.) the original value.

g. Suppose that the resistance of the light bulb located between points D and G is doubled. This would result in the current measured at location G to ____ (increase, decrease, not be affected).

h. Suppose that the resistance of the light bulb located between points D and G is doubled. This would result in the electric potential difference between points D and G to ____ (increase, decrease, not be affected).

i. Suppose that the resistance of the light bulb located between points D and G is doubled. This would result in the current measured at location A to ____ (increase, decrease, not be affected).

j. Suppose that the resistance of the light bulb located between points D and G is doubled. This would result in the current measured at location E to ____ (increase, decrease, not be affected).

k. Suppose that the resistance of the light bulb located between points D and G is doubled. This would result in the current measured at location K to ____ (increase, decrease, not be affected).

Answer: See answers above.

a. - c. Location A is outside or before the branching locations; it represents a location where the total circuit current is measured. This current will ultimately divide into three pathways, with each pathway carrying the same current (since each pathway has the same resistance). Location D is a branch location; one-third of the charge passes through this branch. Location B represents a location after a point at which one-third of the charge has already branched off to the light bulb between points D and G. So at location B, there is two-thirds of the current remaining. And location L is a location in the last branch; so one-third of the charge passes through location L.

d. - f. The current at every branch location and in the total circuit is simply equal to the voltage drop across the branch (or across the total circuit) divided by the resistance of the branch (or of the total circuit). As such, the current is directly proportional to the voltage. So a doubling of the voltage will double the current at every location.

g. The current at a branch location is simply the voltage across the branch divided by the resistance of the branch. So the current at location G is inversely proportional to the resistance of the branch. Doubling the resistance will cause the current to be decreased by a factor of 2.

h. The voltage drop across the first branch (or any branch) is simply equal to the voltage gained by the charge in passing through the battery. For a parallel circuit, the only means of altering a branch voltage drop is to alter the battery voltage.

i. - k. Altering the resistance of a light bulb in a specific branch can alter the current in that branch and the current in the overall circuit. The current in a branch is inversely proportional to the resistance of the branch. So increasing the resistance of a branch will decrease the current of that branch and the overall current in the circuit (as measured at location A). However, the current in the other branches are dependent solely upon the voltage drops of those branches and the resistance of those branches. So while altering the resistance of a single branch alters the current at that branch location, the other branch currents remain unaffected.

Useful Web Links

Parallel Circuits

Part E: Problem-Solving and Circuit Analysis

65. If the current at a given point in a circuit is 2.5 Amps, then how many electrons pass that point on the circuit in a time period of 1 minute.

Answer: 9.375 x 10²⁰ electrons

The current (I) is the rate at which charge passes a point on the circuit in a unit of time. So I = Q/t. Rearranging this equation leads to Q = I•t. Recognizing that a current of 2.5 Amps is equivalent to 2.5 Coulombs per second and that 1 minute is equivalent to 60 seconds can lead to the amount of Coulombs moving pass the point.

Q = I•t = (2.5 C/s)•(60 s) = 150 Coulombs

The charge of a single electron is equal to 1.6 x 10^-19 C. So 150 Coulombs must be a lot of electrons. The actual number can be computed as shown:

# electrons = 150 C • (1 electron / 1.6 x 10^-19 C) = 9.375 x 10²⁰ electrons

Useful Web Links

Electric Current

66. What is the resistance (in ohms) of a typical 40-Watt light bulb plugged into a 120-Volt outlet in your home?

Answer: 360 Ohms

The power dissipated in a circuit is given by the equation P = I•ΔV. Substituting in ΔV/R for the current can lead to an equation relating the resistance (R) to the voltage drop (ΔV) and the power (P).

P = I•ΔV = (ΔV/R)•ΔV = ΔV² / R

Rearrangement of the equation and substitution of known values of power (40 Watts) and voltage (120 V) leads to the following solution.

R = ΔV² / P = (120 V)² / (40 Watts) = 360 Ohms

Useful Web Links

Power Revisited

67. Determine the length of nichrome wire (resistivity value = 150 x 10^-8 ohm•m) required to produce a 1.00 mAmp current if a voltage of 1.5 Volts is impressed across it. The diameter of the wire is 1/16-th of an inch. (GIVEN: 2.54 cm = 1 inch)

Answer: 2.0 x 10³ m

This is clearly an exercise in unit conversion (or at least unit awareness). The resistance (R) of a wire is related to the resistivity (Rho), the length (L) and the cross-sectional area (A) by the equation

R = (Rho) • L / A

This can be rearranged to solve for length

L = R • A / (Rho)

The diameter is given; cross-sectional area is simply given by PI•R². The radius of the wire is one-half the diameter - 1/32 inch.

A = PI•R² = (3.141592) • (1/32 inch) = 0.306796 x10^-3 in²

Since the unit of length for the Rho value is meters, the cross-sectional area will be determined in m² before substitution into the equation. The fact that the conversion involves squared units makes this problem even more trickier. The conversion factors will have to be squared to accomplish the conversion successfully.

A = 0.306796 x10^-3 in² • (2.54 cm / 1 in)² • (1 m / 100 cm)² = 1.979326 x 10^-6 m²

The final quantity which must be determined before computing the wire length is the actual resistance of the wire. Resistance is related to voltage drop (ΔV) and current (I) by the equation R = ΔV / I. Standard units are Ohms, Volts and Amps. Here, the current is given in milliAmps (mAmps); substitution to Amps must be performed before substitution.

R = ΔV / I = (1.5 Volt) / (0.001 Amps) = 1500 Ohms

Now R, A and Rho can be substituted into the length equation to determine the length of the wire in meters.

L = R • A / (Rho) = (1500 Ohms) • (1.979326 x 10^-6 m²) / (150 x 10^-8 ohm•m) = 1.979 x 10³ meters = 2.0 x10³ meters

Useful Web Links

Resistance || Ohm's Law

68. Determine the total monthly cost of using the following appliances/household wires for the given amount of time if each is plugged into a 120-Volt household outlet. The cost of electricity is $0.13 / kW•hr. (Assume that a month lasts for 30 days.)

Appliance (with info from labels)	Time (hours/day)	Power (Watts)	Energy Consumed	Cost ($)
Hair Dryer (12 Amp)	0.10	1440 W	4.32 kW•h	$0.56
Coffee Percolator (9.0 Amp)	0.10	1080 W	3.24 kW•h	$0.42
Light Bulb (100 Watt)	8	100 W	24 kW•h	$3.12
Attic Fan (140 Watt)	10	140 W	42 kW•h	$5.46
Microwave Oven (8.3 Amps)	0.25	946 W	7.47 kW•h	$0.97
Total				$10.53

Answer: See table above.

The power is either explicitly stated (as in the case of the light bulb) or calculated using P = I•ΔV. In this case, the voltage is 120 Volts. The energy consumed is the Power•time. It is useful to express this quantity in the same units for which one is charged for it - kiloWatt • hour. The calculation involves converting power in Watts to kiloWatts by dividing by 1000 and then multiplying by the time in hours/month and then multiplying by 30 days/month. The cost in dollars is simply the kiloWatt•hours of energy used multiplied by the cost of $0.13/kW•hr.

Useful Web Links

Power: Putting Charges to Work || Power Revisited

69. If the copper wire used to carry telegraph signals has a resistance of 10 ohms for every mile of wire, then what is the diameter of the wire. (Given: 1609 m = 1 mile. Resistivity of Cu = 1.7 x 10^-8 ohm•m )

Answer: 0.59 cm

Like Question #67, this is another exercise in unit conversion and unit awareness. The resistance (R) of a wire is related to the resistivity (Rho), the length (L) and the cross-sectional area (A) by the equation

R = (Rho) • L / A

This can be rearranged to solve for cross-sectional area

A = (Rho) • L / R

The area is related to the radius by the equation A = PI•R². The plan will involve determining the Area, then the radius, then the diameter of the wire.

First, note that the given information is: Rho = 1.7 x 10^-8 ohm•m; L = 1 mi = 1609 m; R = 10 Ohm. By substitution, the Area can be determined:

A = (Rho) • L / R = (1.7 x 10^-8 ohm•m) • (1609 m) / (10 Ohm)

A = 2.7353 x 10^-6 m²

The area is in m² units. Since the diameter of wires is typically expressed in centimeters or millimeters, a conversion will be performed. The fact that the conversion involves squared units makes this conversion even more trickier. The conversion factors will have to be squared to accomplish the conversion successfully.

A = 2.7353 x 10^-6 m²• (100 cm )²/ (1 m)² = 0.27353 cm²

Now the area equation (A = PI•R²) can be used to determine the radius.

R = Sqrt (A/PI) = Sqrt [(0.27353 cm² ) / (3.1415)] = Sqrt(8.70673 x 10^-2 cm²)

R = 0.29507 cm

The radius is simple twice the diameter. So d = 0.59014 cm.

Useful Web Links

Resistance || Ohm's Law

70. Determine the resistance of a 1500 Watt electric grill connected to a 120-Volt outlet.

Answer: 9.6 Ohms

P = I•ΔV = (V/R)•ΔV = ΔV² / R

Rearrangement of the equation and substitution of known values of power (1500 Watts) and voltage (120 V) leads to the following solution.

R = ΔV² / P = (120 V)² / (1500 Watts) = 9.6 Ohms

Useful Web Links

Power Revisited

71. Four resistors - 2.0-Ohms, 5.0-Ohms, 12-Ohms and 15-Ohms - are placed in series with a 12-Volt battery. Determine the current at and voltage drop across each resistor.

Answer: See diagram below.

The diagram below depicts the series circuit using schematic symbols. Note that there is no branching, consistent with the notion of a series circuit.

For a series circuit, the overall resistance (R_Tot) is simply the sum of the individual resistances. That is

R_Tot = R₁ + R₂ + R₃ + R₄

R_Tot = 2 ½ + 5 ½ + 12 ½ + 15 ½ = 34 ½

The series of three resistors supplies an overall, total or equivalent resistance of 34 Ohms. Since there is no branching, the current is the same through each resistor. This current is simply the overall current for the circuit and can be determined by finding the ratio of battery voltage to overall resistance (V_Tot/R_Tot).

I_Tot = ΔV_Tot/R_Tot = (12 Volt) / (34 Ohm) = 0.35294 Amps

The current through the battery and through each of the resistors is ~0.353 Amps. The voltage drop across each resistor is equal to the I•R product for each resistor. These calculations are shown below.

ΔV₁ = I₁ • R₁ = (0.35294 Amps) • (2 Ohms) = 0.71 V

ΔV₂ = I₂ • R₂ = (0.35294 Amps) • (5 Ohms) = 1.76 V

ΔV₃ = I₃ • R₃ = (0.35294 Amps) • (12 Ohms) = 4.24 V

ΔV₄ = I₄ • R₄ = (0.35294 Amps) • (15 Ohms) = 5.29 V

Useful Web Links

Series Circuits

72. Four resistors - 2-Ohms, 5-Ohms, 12-Ohms and 15-Ohms - are placed in parallel with a 12-Volt battery. Determine the current at and voltage drop across each resistor.

Answer: See diagram below.

The diagram below depicts the parallel circuit using schematic symbols. Note that there is a branching, consistent with the notion of a parallel circuit.

For a parallel circuit, the reciprocal of overall resistance (1 / R_Tot) is simply the sum of the reciprocals of individual resistances. That is

1 / R_Tot = 1 / R₁ + 1 / R₂ + 1 / R₃ + 1 / R₄

1 / R_Tot = 1 / 2 ½ + 1 / 5 ½ + 1 / 12 ½ + 1 / 15 ½ = 0.850 / ½

R_Tot = 1.17647 ½

The series of three resistors supplies an overall, total or equivalent resistance of ~1.18 Ohms. This total resistance value can be used to determine the total current through the circuit.

I_Tot = ΔV_Tot/R_Tot = (12 Volt) / (1.17647 Ohm) = 10.2 Amps

Since there is branching, the total current will be equal to the sum of the currents at each resistor. The current at each resistor is the voltage drop across each resistor divided by the resistance of each resistor. For series circuits, the voltage drop across each resistor is the same as the voltage gained by the charge in the battery (12 Volts in this case). The branch current calculations are shown below.

I₁ = ΔV₁ / R₁ = (12 Volts) / (2 Ohms) = 6.00 Amp

I ₂ = ΔV₂ / R₂ = (12 Volts) / (5 Ohms) = 2.40 Amp

I ₃ = ΔV₃ / R₃ = (12 Volts) / (12 Ohms) = 1.00 Amp

I ₄ = ΔV₄ / R₄ = (12 Volts) / (15 Ohms) = 0.80 Amp

Useful Web Links

Parallel Circuits

Navigate to:

Review Session Home - Topic Listing

Electric Circuits - Home || Printable Version || Questions with Links

Answers to Questions: All || #1-7 || #8-51 || #52-59 || #60-72

You Might Also Like ...

Users of The Review Session are often looking for learning resources that provide them with practice and review opportunities that include built-in feedback and instruction. If that is what you're looking for, then you might also like the following:

The Calculator Pad

The Calculator Pad includes physics word problems organized by topic. Each problem is accompanied by a pop-up answer and an audio file that explains the details of how to approach and solve the problem. It's a perfect resource for those wishing to improve their problem-solving skills.

Visit: The Calculator Pad Home | Calculator Pad - Electric Circuits
Minds On Physics the App Series

Minds On Physics the App ("MOP the App") is a series of interactive questioning modules for the student that is serious about improving their conceptual understanding of physics. Each module of the series covers a different topic and is further broken down into sub-topics. A "MOP experience" will provide a learner with challenging questions, feedback, and question-specific help in the context of a game-like environment. It is available for phones, tablets, Chromebooks, and Macintosh computers. It's a perfect resource for those wishing to refine their conceptual reasoning abilities. Part 4 of the series includes topics in Electric Circuits.

Visit: MOP the App Home || MOP the App - Part 4

a. one watt; one second	b. one joule; one hour
c. one electron; one second	d. one electron; one hour
e. one volt; one second	f. one volt; one hour
g. one coulomb; one second	h. one coulomb; one hour

a. greater than that through the light bulb	b. less than that through the light bulb
c. the same as that through the light bulb	d. greater than that through each wire
e. less than that through each wire

a. seconds	b. hours
c. years	d. one-millionth of a second
e. one-tenth of a second

a. goes through	b. is expressed across
c. is constant throughout	d. is the rate at which charges move through

a. Voltage	b. Wattage	c. Electric Potential Difference
d. Rate at which charge flows	e. Electric Pressure	f. Energy

a. 1 Ampere = 1 Coulomb / second	b. 1 Joule = 1 Volt / Coulomb
c. 1 Watt = 1 Joule • second	d. 1 Watt = 1 Volt • Coulomb / second
e. 1 Joule / Ohm = 1 Ampere • Coulomb	f. 1 Joule • Ohm = 1 Volt² • second

a. 6	b. 12	c. 24
d. ... nonsense!. The electric potential difference is dependent upon the actual resistance of the resistors

a. increase, increase	b. decrease, decrease
c. increase, decrease	d. decrease, increase
e. increase, remain the same	f. decrease, remain the same
g. remain the same, increase	h. remain the same, decrease
i. remain the same, remain the same

a. less than 4	b. 4	c. more than 4
d. impossible to make such a prediction without knowledge of the resistances.

Electric Potential Comparison	Potential Energy Comparison
V_A = V_B	PE_A = PE _B
V_B > V_C	PE _B > PE _C
V_C = V_D	PE _C = PE _D
V_D > V_E	PE _D > PE _E
V_E = V_F	PE _E = PE _F
V_F > V_G	PE _F > PE _G
V_G = V_H	PE _G = PE _H
V_H < V_A	PE _H < PE _A