Relativistic Length Contraction
One of the peculiar aspects of Einstein's theory of special relativity is that the length of objects moving at relativistic speeds undergoes a contraction along the dimension of motion. An observer at rest (relative to the moving object) would observe the moving object to be shorter in length. That is to say, that an object at rest might be measured to be 200 feet long; yet the same object when moving at relativistic speeds relative to the observer/measurer would have a measured length which is less than 200 ft. This phenomenon is not due to actual errors in measurement or faulty observations. The object is actually contracted in length as seen from the stationary reference frame. The amount of contraction of the object is dependent upon the object's speed relative to the observer.
The animations below depict this phenomena of length contraction. In each animation a spaceship is moving past Earth at a high speed. The spaceship would be measured to be 200 feet in length when at rest relative to the observer.
Spaceship Moving at the 10 % the Speed of Light
Spaceship Moving at the 86.5 % the Speed of Light
Spaceship Moving at the 99 % the Speed of Light
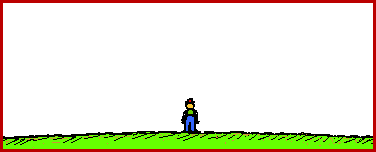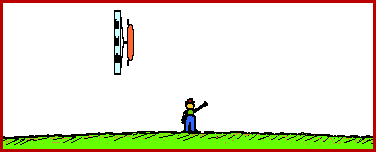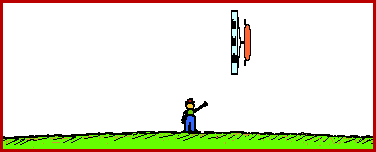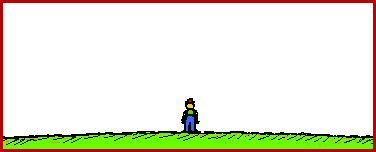
Spaceship Moving at the 99.99 % the Speed of Light
Note that the length contraction is only significant when the object is moving at relativistic speeds - i.e., speeds which are a significant fraction of the speed of light. Furthermore, note that the contraction only occurs in the dimension of the object's motion. If the object is moving horizontally, then it is the horizontal dimension which is contracted; there would be no contraction of the height of the object. This information is summarized in the table below.
|
Speed of Spaceship |
Observed Length |
Observed Height |
|
At rest |
200 ft |
40 ft |
|
10 % the speed of light |
199 ft |
40 ft |
|
86.5 % the speed of light |
100 ft |
40 ft |
|
99 % the speed of light |
28 ft |
40 ft |
|
99.99 % the speed of light |
3 ft |
40 ft |