The Cart and The Brick

Part B

Collisions between objects are governed by laws of momentum and energy. When a collision occurs in an isolated system, the total momentum of the system of objects is conserved. Provided that there are no net external forces acting upon the objects, the momentum of all objects before the collision equals the momentum of all objects after the collision. If there are only two objects involved in the collision, then the momentum lost by one object equals the momentum gained by the other object.

The animation below portrays the collision between a 1.0-kg cart and a 2-kg dropped brick. It will be assumed that there are no net external forces acting upon the two objects involved in the collision. The only net force acting upon the two objects (the cart and the dropped brick) are internal forces - the force of friction between the cart and the dropped brick. The before- and after-collision velocities and momentum are shown in the data tables.

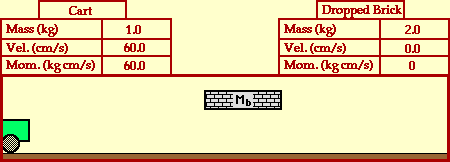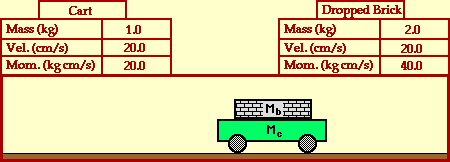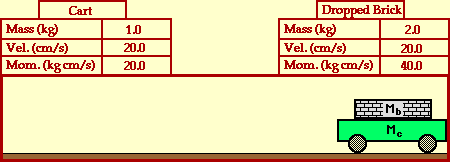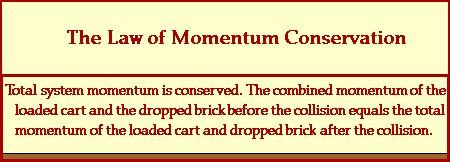
In the collision between the cart and the dropped brick, total system momentum is conserved. Before the collision, the momentum of the cart is 60 kg*cm/s and the momentum of the dropped brick is 0 kg*cm/s; the total system momentum is 60 kg*cm/s. After the collision, the momentum of the cart is 20.0 kg*cm/s and the momentum of the dropped brick is 40.0 kg*cm/s; the total system momentum is 60 kg*cm/s. The momentum of the cart-dropped brick system is conserved. The momentum lost by the cart (40 kg*cm/s) is gained by the dropped brick.

For more information on physical descriptions of motion, visit The Physics Classroom Tutorial. Detailed information is available there on the following topics: